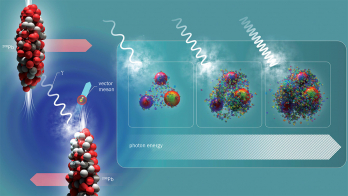
Jefferson Laboratory researchers discuss two new models of spin transfer.
A continuing mystery in nuclear and particle physics is the large polarization observed in the production of Λ hyperons in high-energy, proton–proton interactions. These effects were first reported in the 1970s in reactions at incident proton momenta of several hundred GeV/c, where experiments measured surprisingly strong hyperon polarizations of around 30% (Heller 1997). Although the phenomenology of these reactions is now well known, the inability to distinguish between various competing theoretical models has hampered the field (Zuo-Tang and Boros 2000).

Image credit: Jefferson Lab.
Two new measurements from the US Department of Energy’s Jefferson Lab in Virginia are now challenging existing ideas on quark spin dynamics through studies of beam-recoil spin transfer in the electro- and photoproduction of K+Λ final states from an unpolarized proton target. Analyses of the two experiments in Hall B at Jefferson Lab using the CLAS spectrometer (figure 1) have provided extensive results of spin transfer from the polarized incident photon (real or virtual) to the final state Λ hyperon.
The results indicate that the Λ polarization is predominantly in the direction of the spin of the incoming photon, independent of the centre-of-mass energy or the production angle of the K+. Moreover, the photoproduction data show that, even where the transferred Λ polarization component along the photon direction is less than unity, the total magnitude of the polarization vector is equal to unity. Since these observations are not required by the kinematics of the reaction (except at extreme forward and backward angles) there must be some underlying dynamical origin.

Both analyses have proposed simple quark-based models to explain the phenomenology, however they differ fundamentally in their description of the spin transfer mechanism. In the electroproduction analysis a simple model has been proposed from data using a 2.567 GeV longitudinally polarized electron beam (Carman et al. 2003). In this case a circularly polarized virtual photon (emitted by the polarized electron) strikes an oppositely polarized u quark inside the proton (figure 2a). The spin of the struck quark flips in direction according to helicity conservation and recoils from its neighbours, stretching a flux-tube of gluonic matter between them. When the stored energy in the flux-tube is sufficient, the tube is “broken” by the production of a strange quark–antiquark pair (the hadronization process).
In this simple model, the observed direction of the Λ polarization can be explained if it is assumed that the quark pair is produced with two spins in opposite directions – anti-aligned – with the spin of the s quark aligned opposite to the final u quark spin. The resulting Λ spin, which is essentially the same as the s quark spin, is predominantly in the direction of the spin of the incident virtual photon. The spin anti-alignment of the ss pair is unexpected, because according to the popular 3P0 model, the quark–antiquark pair should be produced with vacuum quantum numbers (J = 0, S = 1, L = 1, i.e. Jπ = 0+), which means that their spins should be aligned two-thirds of the time (Barnes 2002). This could imply that this model for hadronization may not be as widely applicable as previously thought.
The new photoproduction analysis, with data using a circularly polarized real photon beam in the 0.5–2.5 GeV range, introduces a different model that can also explain the Λ polarization data. In this hypothesis, shown in figure 2b, the strange quark–antiquark pair is created in a 3S1 configuration (J = 1, S = 1, L = 0, i.e. Jπ = 1–). Here, following the principle of vector-meson dominance, the real photon fluctuates into a virtual φ meson that carries the polarization of the incident photon. Therefore, the quark spins are in the direction of the spin of the photon before the hadronization interaction.
The s quark of the pair merges with the unpolarized di-quark within the target proton to form the Λ baryon. The s quark merges with the remnant u quark of the proton to form a spinless K+ meson. In this model, the strong force, which rearranges the s and s quarks into the Λ and K+, respectively, can precess the spin of the s quark away from the beam direction, but the s quark, and therefore the Λ, remains 100% polarized. This provides a natural explanation for the observed unit magnitude of the Λ polarization vector seen for the first time in the measurements by CLAS.
The model interpretations presented from the two viewpoints do not necessarily contradict each other. Both assume that the mechanism of spin transfer to the Λ hyperon involves a spectator Jπ = 0+ di-quark system. The difference is in the role of the third quark. Neither model specifies a dynamical mechanism for the process, namely the detailed mechanism for quark-pair creation in the first case or for quark spin precession in the second. If we take the gluonic degrees of freedom into consideration, the model proposed in the electroproduction paper (Carman et al. 2003) can be realized in terms of a possible mechanism in which a colourless Jπ = 0– two-gluon subsystem is emitted from the spectator di-quark system and produces the ss pair (figure 2a). This is in conflict with the 3P0 model, which requires a Jπ= 0+ exchange. To the same order of gluon coupling, the model interpretation proposed by the photoproduction analysis (Schumacher 2007) is the quark-exchange mechanism, which is again mediated by a two-gluon current. The amplitudes corresponding to these models may both be present in the production, in principle, and contribute at different levels depending on the reaction kinematics.
Extending these studies to the K*+Λ exclusive final state should be revealing. In the electroproduction model, the spin of the u quark is unchanged when switching from a pseudoscalar K+ to a vector K*+. If the ss quark pair is produced with anti-aligned spins, the spin direction of the Λ should flip. On the other hand, in the photoproduction model the u quark in the kaon is only a spectator. Changing its spin direction – changing the K+ to a K*+ – should not change the Λ spin direction. Thus, there are ways to disentangle the relative contributions and to understand better the reaction mechanism and dynamics underlying the associated strangeness-production reaction. Analyses at CLAS are underway to extract the polarization transfer to the hyperon in the K*+Λ final state.
Beyond the studies of hyperon production, understanding the dynamics in a process of this sort can shed light on quark–gluon dynamics in a domain thought to be dominated by traditional meson and baryon degrees of freedom. These issues are relevant for a better understanding of strong interactions and hadroproduction in general, owing to the non-perturbative nature of QCD at these energies. We eagerly await further experimental studies and new theoretical efforts to understand which multi-gluonic degrees of freedom dominate in quark pair creation and their role in strangeness production, as well as the appropriate mechanism(s) for the dynamics of spin transfer in hyperon production.