One of the most remarkable discoveries of recent years is that the universe appears to be dominated by some form of “dark energy”, as Eric Linder explains.
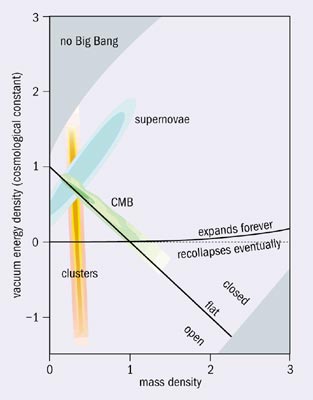
Cosmology has recently achieved its version of a standard model, called the “cosmic concordance”. This gives a broad picture of the components in the universe within the strongly tested framework of the hot Big Bang model. Of these components, only about 4% amount to the familiar baryons of the Standard Model of particle physics, and even some of these are “dark” or not evident directly from the light of distant objects. Another 20-25% is nonbaryonic dark matter, presumably either weakly interacting massive particles or axions, theorized elements of high-energy physics. But the majority of the energy density, some 70-75%, is detected only through its effect of accelerating the global expansion of the universe. This background energy, which is smooth out to scales larger than that of any matter structures such as clusters of galaxies, is named “dark energy”.
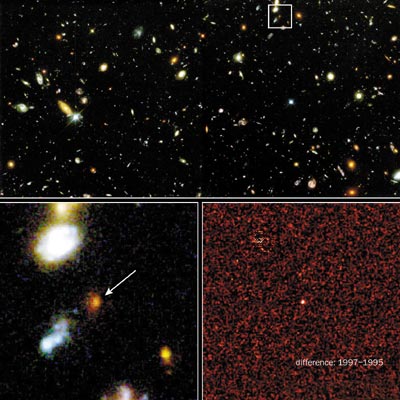
Dark energy was first discovered in 1998 by two groups using supernovae as markers of cosmological distance as a function of time – the Supernova Cosmology Project led by Saul Perlmutter at Lawrence Berkeley National Laboratory and the High-z Supernova Search Team led by Brian Schmidt at Australian National University. Measurements indicated that distant supernovae were dimmer than expected from the cosmological inverse square law in a universe dominated by matter (S Perlmutter et al. 1999, A Riess et al. 1998). That is, they appeared to be further away than expected from the expansion rate of the universe if gravitation due to the matter contents were the main force. Some form of dark energy was required at the 99% confidence level, and in amounts sufficient to counteract, on cosmic scales, the gravitational attraction from the clustered matter.
Since then, deeper and more precise supernova measurements and further lines of evidence confirm this conclusion (J Tonry et al. 2003, R Knop et al. 2003, D Spergel et al. 2003). Detailed measurements of the cosmic microwave background power spectrum, by the Wilkinson Microwave Anisotropy Probe satellite and by ground-based experiments, imply the presence of dark energy too. They also show that the spatial geometry of the universe is consistent with the flatness prediction of inflation. But observations of galaxy clusters tell us that the matter contribution to the total energy density can amount to only 20-30% of the needed critical density. Any two of the three lines of evidence imply that the dark energy composes roughly three-quarters of the energy density of the universe, while the third method provides a crosscheck. Such an amount of dark energy acts to accelerate the cosmic expansion.
The nature of dark energy
While gravitation due to matter or radiation is attractive, a sufficiently negative pressure p would offset a positive energy density ρ to give repulsive gravity under Einstein’s equations (the gravitating density depends on ρ+3p), pulling on space to accelerate the expansion of the universe. Researchers often discuss this in terms of the equation of state ratio of the pressure to energy density: w = p/ρ.
Negative pressures are not a wholly exotic phenomenon. After all, one of the equations of expansion of the universe, the Friedmann equation, looks remarkably similar to the first law of thermodynamics: d(ρV) = -pdV, where V is the volume considered. Negative pressure leads to an overall plus sign, turning this equation into something that looks like the tension in a spring or rubber band. Such a “springiness” of space was postulated soon after Einstein developed the general theory of relativity in his cosmological constant term, and Hermann Weyl attempted to link such a background energy to the quantum vacuum. If the vacuum is a true ground state then all observers must agree on its form. But the only Lorentz invariant energy-momentum tensor is the diagonal Minkowski tensor that has negative pressure equal and opposite to its energy density, that is, the cosmological constant has equation of state ratio w = -1. This would cause an accelerating universe.
So why are cosmologists not satisfied with identifying the cosmological constant with dark energy? In The Hunting of the Snark – the poem by Lewis Carroll, who was in fact Charles Dodgson, a mathematician at Oxford – when the explorers set sail to find the mysterious snark, the captain “had bought a large map representing the sea, without the least vestige of land: and the crew were much pleased when they found it to be a map they could all understand.” The cosmological constant term is such a featureless sea, but there are two problems with using it to describe our universe. The expected sea level for the quantum vacuum is much higher than we observe: naively one should indeed have a featureless universe, with matter drowned by 120 orders of magnitude below the energy density of the cosmological constant. But the cosmic concordance measures only a factor of a few difference. Furthermore, the matter and radiation we see in the universe evolves with the expansion of the universe, while the cosmological constant does not. Even an order of magnitude equality between them occurs in only one characteristic timescale (e-folding), out of the 23 in the expansion of the universe since the well-understood epoch of nuclei formation in the early universe. (See S Weinberg 1989 and S Carroll 2001 for more on these fine-tuning and coincidence puzzles.)
Hunting the dark energy
Researchers are thus driven to consider other explanations for the dark energy. Models with dynamical high-energy physics fields, often called “quintessence” when involving a simple scalar field, go some way toward alleviating the timing or coincidence puzzle, though there is still no clear underlying theory explaining the current effective energy density. Such a field would need an effective mass of 10-33 eV, that is, with a Compton wavelength of the order of the radius of the universe. However, there are rich attempts at phenomenology stretching back two decades (longer if scalar-tensor theories of gravitation are included). An early high-energy physics model was proposed by Andrei Linde in 1986, demonstrating how a linear potential could give rise to accelerating expansion. On the cosmology side, Robert Wagoner in 1986 examined how a general equation of state component would not only affect the expansion, but could be observationally probed with cosmological distance and age measurements.
Both the modelling of and the investigation of the observational consequences of dark energy are now active industries within research in cosmology, covering a wide variety of the physics of the early and late universe. In general, the dark-energy equation of state will vary with time and so needs to be probed with observations over a range of epochs, or astronomical redshifts z (the fractional difference in the scale of the universe today relative to an earlier time). The major challenge over the next few years in cosmology will be to characterize the equation of state function w (z ). On the phenomenology front, one might hope for a natural, robust model to emerge, but the theorists’ prolificness seems too great for this to settle the question. Indeed, models beyond scalar fields involving modifications of general relativity, extra dimensions, or quantum-phase transitions have also been proposed. Fortunately these can be written in terms of an effective w (z ) (E Linder and A Jenkins 2003) and subjected to cosmological measurements.
Three main routes to probing dark energy exist in cosmology. The first, and currently most favoured, involves mapping the expansion history of the universe. The second seeks to measure the growth rate of the formation of large-scale structures such as clusters of galaxies. The third involves the cosmic microwave background radiation – looking not for the time variation of the dark energy (since the cosmic microwave background photons effectively all come from the same redshift), but for the subtle spatial fluctuations in the dark-energy distribution on cosmic scales. Observations of Type Ia supernovae, which first discovered the dark energy in 1998, fall in the first category and seem the most promising. The second and third approaches are likely to run into limits imposed, respectively, by uncertainties involving entangled astrophysics and cosmic variance (intrinsic uncertainty due to observing only one universe). However, new methods and cross correlations between probes may eventually be practical.
In mapping the expansion history, cosmologists probe the deceleration due to the gravitation of matter and the acceleration due to dark energy at various epochs. Variations in the growth of distances reveal a picture of the cosmic environment, and hence the dynamic influence of dark energy, in the way that the width of tree rings indicates the Earth’s climatic environment over time. Type Ia supernovae can be seen to great distances and calibrated in luminosity (made “standard candles” through detailed observations). Thus the measurement of the received flux directly indicates their distance, and hence the time in the past they exploded, while the redshift of the photons is simply the ratio of the size of the universe now relative to then. Together these give the exact expansion history.
Future endeavours
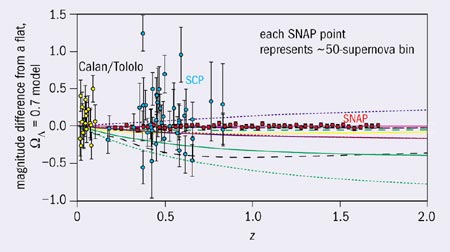
The best current supernova data extend out only to redshift z ≅ 1 (when the scale of the universe was 1/(1+z ) = 1/2 its current size) with any reasonable statistics, but they already constrain the averaged equation of state ratio to w = -1.05+0.15-0.20 (R Knop et al. 2003) or w = -1.0+0.14-0.24 (J Tonry et al. 2003). Clues to the underlying physical theory, however, reside in the dynamics, the time-varying function w (z ). A dedicated dark-energy mission, the Supernova/Acceleration Probe (SNAP) satellite, is being designed to determine the present value w0 to 7% and derivative w ‘ = dw/dz to ±0.15 (1 σ, including both statistical and systematic uncertainties). Led by Michael Levi and Saul Perlmutter of the Lawrence Berkeley National Laboratory, the project involves over 100 scientists and engineers from more than 15 institutions, including France and Sweden. Launch is proposed for 2010.
Meanwhile, an intense research effort continues. One example is the European Dark Energy Network (EDEN), a proposed European Union research training network of 13 nodes (including CERN, led by Gabriele Veneziano), coordinated by Pedro Ferreira of Oxford. Models attempt to link dark energy to dark matter, extra dimensions, modifications of gravity and a zoo of simple and non-minimally coupled scalar fields. These predict a range of values for the equation of state ratio w0, within the current constraints, and a wholly open variety of w ‘, both positive and negative. Some even lead to an eventual reversal of the acceleration and a collapse of the universe. It is amusing that the first dark-energy model, the linear potential, possesses this quality. Future data will constrain the allowed parameters of classes of high-energy physics models and the fate of the universe, including how long we have left until a cosmic doomsday! (See R Kallosh et al. 2003 for the linear potential case leading to a Big Crunch and R Caldwell et al. 2003 for a Big Rip.)

Can signs of the nature of dark energy be uncovered at particle accelerators? It is difficult to see how. The energy scale of the physics is presumably of the order 1016 GeV, and by its “dark” nature the coupling to matter is vanishingly small. On scales smaller than the universe, the dynamical effect of dark energy is negligible. The entire dark-energy content within the solar system equals that of three hours of solar luminosity. Perhaps if the physics involves the modification of gravity or extra dimensions, a precise laboratory test could see a signature (see E Adelberger et al. 2003 for a current experiment). But the true hunting grounds for the nature of dark energy and the physics causing the acceleration of the universe lie in cosmology. Just as advances have been made in the past two decades in theory and observations beyond the simplistic view of early universe inflation as a pure deSitter phase – “sea without the least vestige of land” – so too will dark-energy studies delve deeper into fundamental physics. Instruments now being designed could tell us within the next decade whether we must come to grips with a minuscule but finite cosmological constant or some exciting new dynamical physics.
Further reading
E G Adelberger et al. 2003 Ann. Rev. Nucl. Part. Sci. 53 (www.arxiv.org/abs/hep-ph/0307284).
S W Allen, R W Schmidt and A C Fabian 2002 MNRAS 334 L11.
R R Caldwell, M Kamionkowski and N N Weinberg 2003 (submitted) Phys. Rev. Lett. (astro-ph/0302506).
S Carroll 2001 Living Rev. Rel. 4 1.
R Kallosh et al. 2003 (submitted) JCAP (astro-ph/0307185).
R Knop et al. 2003 (in press) Ap. J.
A Linde 1987 Three hundred years of gravitation ed. S W Hawking and W Israel, 604.
E V Linder, A Jenkins 2003 (in press) MNRAS (astro-ph/0305286).
S Perlmutter et al. 1999 Ap. J. 517 565.
A Riess et al. 1998 Astron. J. 116 1009.
D N Spergel et al. 2003 (in press) Ap. J. (astro-ph/0302209).
J Tonry et al. 2003 (in press) Ap. J. (astro-ph/0305008).
R V Wagoner 1986 (unpublished lecture notes).
S Weinberg 1989 Rev. Mod. Phys. 61 1.
J Weller and A Albrecht 2002 Phys. Rev. D65 103512.





