The latest generation of high-current electron accelerators in the GeV energy range is breathing new life into a variety of investigations based on the photoproduction of kaons, as Terry Mart explains.
Since the discovery of strangeness by Murray Gell-Mann and Kazuhiko Nishijima almost five decades ago, interest in this degree of freedom has remained alive, with investigations now spanning the range from quarks to nuclei. For nuclei in particular, strangeness has given experimenters a new tool for probing all nuclear levels while avoiding “Pauli blocking”, which is an inherent problem for conventional nuclei where low levels are already filled with neutrons and protons. Hypernuclear systems are therefore very promising subjects of study in nuclear physics, and there have been considerable efforts in theory and experiment alike to uncover the behaviour of nuclei “doped” with one or several hyperons.
Hypernuclei can also be produced using electromagnetic processes, such as kaon photoproduction off a nucleus. Compared with the conventional hadronic mechanism, electromagnetic production of hypernuclei has a clear advantage: since the photon and kaon interact weakly with the nucleus, the process can occur deep inside the nuclear interior. Furthermore, electromagnetic processes are fully controllable in comparison with hadronic ones, so the photoproduction of a kaon plus a hypernucleus provides a clean way to study hypernuclear spectra. In addition, the associated production off a deuteron can be used to study the hyperon-nucleon interaction in the final state, while the quasi-free kaon photoproduction off nuclei serves as an important tool for investigating kaon-nucleus and hyperon-nucleus optical potentials.
While the history of kaon photoproduction off the nucleon goes back to the mid-1950s, a more serious phenomenological study was begun in 1966 by H Thom at Cornell (Thom 1966). Extensive investigations continued until the early 1970s, but then interest temporarily declined, mainly due to the lack of experimental facilities. The field was revived in the late 1980s after the construction of a new generation of high duty-factor electron accelerators providing continuous, high-current and polarized beams in the energy region of a few GeV. Now, the operation of these accelerators, such as ELSA in Bonn, MAMI in Mainz, CEBAF at the Jefferson Laboratory in Virginia, GRAAL in Grenoble and Spring-8 in Osaka, has boosted efforts to advance our knowledge in the strangeness sector through the electromagnetic production of kaons. Recently, there has been a great deal of excitement concerning the detection of a five-quark state – the “pentaquark” – in kaon photoproduction at ELSA, CEBAF and Spring-8. and while it is clear that kaon electroproduction contains a lot of rich information, this article will concentrate on several other aspects that make kaon photoproduction an up-to-date research topic.
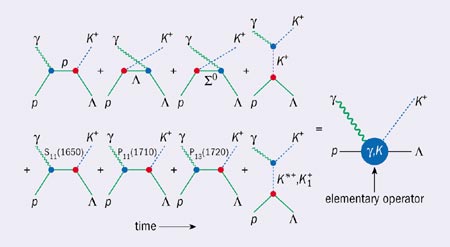
Kaon photoproduction processes cannot be properly understood without an elementary production operator that describes the production mechanism off the nuclear constituents (proton or neutron). At the elementary level, the investigation is most effectively performed in the framework of an isobar approach based on nucleons and hyperons – that is, the corresponding operator is constructed from the Feynman diagrams shown in figure 1. (Quark models can also be used but they are beyond the scope of this article.) Furthermore, the isobar approach has the advantage that it has several simple phenomenological applications, as discussed later.
As can be seen from figure 1, several “ingredients” enter the elementary photoproduction operator via intermediate particles. Among them, the most crucial are: the number of meson and baryon resonances participating in the reaction, the properties of those resonances, hadronic and electromagnetic vertex factors, and methods to maintain fundamental symmetries of the operator.
Understanding these ingredients from first principles is currently beyond our capabilities, and this leads to some intrinsic problems on the theoretical side. The best we can do in the mean time is to restrict the number of resonances in the model to as few as possible by following the recommendation from the Particle Data Book, that is, including only the resonances that have significant decay widths to kaon channels, and fitting all unknown factors or constants (Hagiwara et al. 2002). Although this procedure may seem too pragmatic, the result shows good agreement with experimental data and has a wide range of applications in processes involving kaons and hyperons.
The search for ‘missing’ resonances
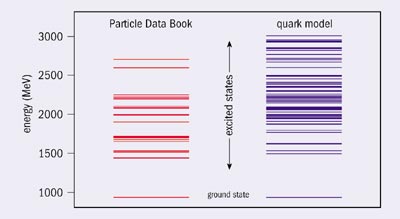
The physics of nucleon-resonance excitation continues to provide a major challenge to hadronic physics due to the nonperturbative nature of quantum chromodynamics (QCD) at these energies. While methods such as chiral perturbation theory are not amenable to N* physics, lattice QCD has only recently begun to contribute to this field. Thus, most of the theoretical work on the nucleon excitation spectrum has been performed in the realm of quark models. Interestingly, these models predict a much richer resonance spectrum than has been observed in πN → πN scattering experiments (see figure 2), the main source of the Particle Data Book. The obvious question is: where are the other resonances? One may argue that perhaps they are hiding behind some prominent resonances and we need better resolution to single them out. On the other hand, quark models themselves have suggested that those “missing” resonances may couple strongly to other channels, such as the Λ and Σ channels.
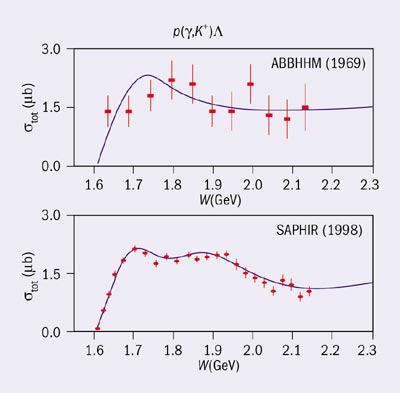
Recently, much improved data have become available in the γp → K+Λ, γp → K+Σ0 and γp → K0Σ+ channels, from total cross-sections to polarization observables. The new SAPHIR total cross-section data for the γp → K+Λ channel, shown in figure 3, indicate for the first time a structure around a centre-of-mass energy of W = 1.9 GeV. This structure could not be resolved before due to the low quality of the old data. Indeed, these new data can guide us to select the most important resonances in this process. Cornelius Bennhold and I have interpreted this structure as the evidence for the missing resonance D13(1895) (Mart and Bennhold 2000). This was previously predicted by a constituent quark model to have a mass of 2080 MeV (Capstick and Roberts 1994). Although we found a remarkable agreement between the predicted and the extracted photocouplings, firmer evidence awaits rigorous calculations using a coupled-channels approach.
Searching for “missing” resonances is not only the business of studies in kaon-hyperon production – several other studies have tried to find these resonances in vector meson production. A new organization, BRAG (Baryon Resonance Analysis Group), has been set up to form a network between researchers working in this field and to optimize the available resources. More than 100 physicists from 18 countries have now joined this network. Clearly, this field will attract more attention from the hadronic physics community around the world in the coming years.
From deuterons to hypernuclei
The electromagnetic production of kaons can also be performed off a deuteron target, where one of the nucleons inside the deuteron absorbs the photon and transforms into a kaon and a Λ hyperon, as shown in figure 4a. Since a Λ cannot bind to a nucleon, photoproduction leads to a break-up process. However, before the hyperon leaves the nucleon, both particles interact strongly. Thus, a phenomenological description of this process requires information on the hyperon-nucleon (YN) potential; or in other words, this process paves the way for investigating the YN potential via electromagnetic processes.
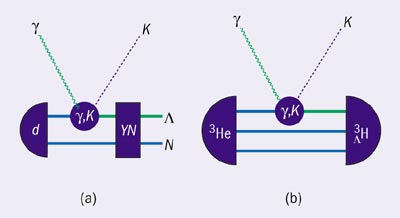
Contrary to the case of the nucleon-nucleon (NN) potential, the properties of the hyperon-nucleon interaction are still somewhat uncertain. In the case of NN forces, one has the rich set of NN scattering data at one’s disposal to adjust NN force parameters. This set is essentially absent in the case of the YN system, since performing YN scattering experiments is very difficult. Kaon photoproduction off the deuteron is therefore well suited to tackle this problem by testing various available models for the YN potential. Such a study has been performed by Hisahiko Yamamura and colleagues (Yamamura et al. 2000). By using the modern Nijmegen soft-core YN potential, they found sizeable final-state effects caused by this potential near K+ΛN and K+ΣN thresholds.
If kaon photoproduction is performed off a helium nucleus (a helion), then a hypertriton is formed in the final state (see figure 4b). The electromagnetic production of the hypertriton is a clean mechanism for studying this lightest hypernucleus. The production is expected to act as a complementary tool for investigating hypernuclear spectra. In a study using realistic 3He and hypertriton wave functions, obtained as solutions of Faddeev equations, we have found that Fermi motions in the nucleus play a significant role in this process and the cross-section is of the order of several nanobarns (Mart et al. 1998). By extending these investigations to heavier nuclei, we could eventually cover the hypernuclear chart.
Inside the nucleon
The internal structure of the nucleon is responsible for its ground-state properties, such as hadronic and electromagnetic form factors and the anomalous magnetic moment, while at higher energies this finite internal structure creates a series of resonances in the mass region of 1-2 GeV, as shown in figure 2. The ground-state properties and the resonance spectra are not all independent phenomena, however; they are related by a number of sum rules. One of these is the Gerasimov-Drell-Hearn (GDH) sum rule (see equation 1), which connects the magnetic moments of the nucleons and their helicity structures in the resonance region. The derivation of this sum rule is based on general principles: Lorentz and gauge invariance, crossing symmetry, causality and unitarity. The only assumption in deriving equation 1 is that the scattering amplitude goes to zero in the limit, photon energy ν→∞, thus there is no subtraction hypothesis.

Although the GDH sum rule was proposed more than 30 years ago, no direct experiment has been performed to investigate whether or not the sum rule converges. However, with the advent of the new high-intensity and continuous-electron-beam accelerators, accurate measurements of the right-hand side of equation 1, as well as contributions from individual final states, are now possible.
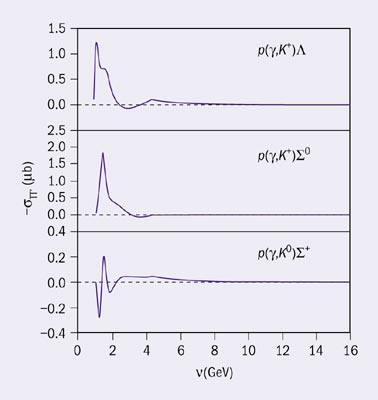
The contribution from kaon-hyperon final states is of particular interest because strange quarks are explicitly present in the final states. Using the elementary operator obtained from figure 1, calculations of the contribution for photon energy up to 2.2 GeV show that about 3.5% of the nucleon magnetic moment comes from the strange quark contribution (Sumowidagdo and Mart 1999). Very recently, a refined calculation up to about 16 GeV (see figure 5) has yielded a relatively smaller value of 1.25%, which is due to the oscillating behaviour of the cross-section (Mart and Wijaya 2003). Despite these small values, however, the calculations indicate that contributions from strangeness to the magnetic moment of proton is obviously significant. It is a challenge to the experimenters to confront the prediction shown in figure 5 with their future data. There is still much of interest to study in kaon photoproduction.
Further reading
S Capstick and W Roberts 1994 Phys. Rev. D49 4570.
K Hagiwara et al. 2002 Phys. Rev. D66 010001.
T Mart et al. 1998 Nucl. Phys. A640 235.
T Mart and C Bennhold 2000 Phys. Rev. C61 012201.
T Mart and T Wijaya 2003 Acta Phys. Polon. B34 2651.
S Sumowidagdo and T Mart 1999 Phys. Rev.C60 028201.
H Thom 1966 Phys. Rev. 151 1322.
H Yamamura et al. 2000 Phys. Rev. C61 014001.