Strong interactions

Murray Gell-Mann’s scientific career began at the age of 15, when he received a scholarship from Yale University that allowed him to study physics. Afterwards he went to the Massachusetts Institute of Technology and worked under Victor Weisskopf. He completed his PhD in 1951, at the age of 21, and became a postdoc at the Institute for Advanced Study in Princeton.

The following year, Gell-Mann joined the research group of Enrico Fermi at the University of Chicago. He was particularly interested in the new particles that had been discovered in cosmic rays, such as the six hyperons and the four K-mesons. Nobody understood why these particles were created easily in collisions of nucleons, yet decayed rather slowly. To understand the peculiar properties of the new hadrons, Gell-Mann introduced a quantum number, which he called strangeness (S): nucleons were assigned S = 0; the Λ hyperon and the three Σ hyperons were assigned S = (–1); the two Ξ hyperons had S = (–2); and the negatively charged K-meson had S = (–1).

Strange assumptions

Gell-Mann assumed that the strangeness quantum number is conserved in the strong and electromagnetic interactions, but violated in the weak interactions. The decays of the strange particles into particles without strangeness could only proceed via the weak interaction.

The idea of strangeness thus explained, in a simple way, the production and decay rates of the newly discovered hadrons. A new particle with S = (–1) could be produced by the strong interaction together with a particle with S = (+1) – e.g. a negatively charged Σ can be produced together with a positively charged K meson. However, a positively charged Σ could not be produced together with a negatively charged K meson, since both particles have S = (–1).

In 1954 Gell-Mann and Francis Low published details of the renormalisation of quantum electrodynamics (QED). They had introduced a new method called the renormalisation group, which Kenneth Wilson (a former student of Gell-Mann) later used to describe the phase transitions in condensed-matter physics. Specifically, Gell-Mann and Low calculated the energy dependence of the renormalised coupling constant. In QED the effective coupling constant increases with the energy. This was measured at the LEP collider at CERN, and found to agree with the theoretical prediction.

In 1955 Gell-Mann went to Caltech in Pasadena, on the invitation of Richard Feynman, and was quickly promoted to full professor – the youngest in Caltech’s history. In 1957, Gell-Mann started to work with Feynman on a new theory of the weak interaction in terms of a universal Fermi interaction given by the product of two currents and the Fermi constant. These currents were both vector currents and axial-vector currents, and the lepton current is a product of a charged lepton field and an antineutrino field. The “V–A” theory showed that since the electrons emitted in a beta-decay are left-handed, the emitted antineutrinos are right-handed – thus parity is not a conserved quantum number. Some experiments were in disagreement with the new theory. Feynman and Gell-Mann suggested in their paper that these experiments were wrong, and it turned out that this was the case.

In 1960 Gell-Mann invented a new symmetry to describe the new baryons and mesons found in cosmic rays and in various accelerator experiments. He used the unitary group SU(3), which is an extension of the isospin symmetry based on the group SU(2). The two nucleons and the six hyperons are described by an octet representation of SU(3), as are the eight mesons. Gell-Mann often described the SU(3)-symmetry as the “eightfold way” in reference to the eightfold path of Buddhism. At that time, it was known that there exist four Δ resonances, three Σ resonances and two χ resonances. There is no SU(3)-representation with nine members, but there is a decuplet representation with 10 members. Gell-Mann predicted the existence and the mass of a negatively charged 10th particle with strangeness S = (–3), which he called the Ω particle.

The Ω is unique in the decuplet: due to its strangeness it could only decay by the weak interaction, and so would have a relatively long lifetime. This particle was discovered in 1964 by Nicholas Samios and his group at Brookhaven National Laboratory, at the mass Gell-Mann had predicted. The SU(3) symmetry was very successful and in 1969 Gell-Mann received the Nobel Prize in Physics “for his contributions and discoveries concerning the classification of elementary particles and their interactions.”

In 1962 Gell-Mann proposed the algebra of currents, which led to many sum rules for cross sections, such as the Adler sum rule. Current algebra was the main topic of research in the following years and Gell-Mann wrote several papers with his colleague Roger Dashen on the topic.

Quark days

In 1964 Gell-Mann discussed the triplets of SU(3), which he called “quarks”. He proposed that quarks were the constituents of baryons and mesons, with fractional electric charges, and published his results in Physics Letters. Feynman’s former PhD student George Zweig, who was working at CERN, independently made the same proposal. But the quark model was not considered seriously by many physicists. For example, the Ω is a bound state of three strange quarks placed symmetrically in an s-wave, which violated the Pauli principle since it was not anti-symmetric. In 1968, quarks were found indirectly in deep-inelastic electron–proton experiments performed at SLAC.

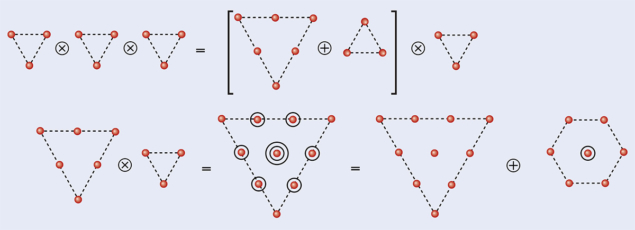
By then it had been proposed, by Oscar Greenberg and by Moo-Young Han and Yoichiro Nambu, that quarks possess additional properties that keep the Pauli principle intact. By imagining the quarks in three “colours” – which later came to be called red, green and blue – hadrons could be considered as colour singlets, the simplest being the bound states of a quark and an antiquark (meson) or of three quarks (baryon). Since baryon wave functions are antisymmetric in the colour index, there is no problem with the Pauli principle. Taking the colour quantum number as a gauge quantum number, like the electric charge in QED, yields a gauge theory of the strong interactions: colour symmetry is an exact symmetry and the gauge bosons are massless gluons, which transform as an octet of the colour group. Nambu and Han had essentially arrived at quantum chromodynamics (QCD), but in their model the quarks carried integer electrical charges.

The quark model was not considered seriously by many physicists

I was introduced to Gell-Mann by Ken Wilson in 1970 at the Aspen Center of Physics, and we worked together for a period. In 1972 we wrote down a model in which the quarks had fractional charges, proposing that, since only colour singlets occur in the spectrum, fractionally charged quarks remain unobserved. The discovery in 1973 by David Gross, David Politzer and Frank Wilczek that the self-interaction of the gluons leads to asymptotic freedom – whereby the gauge coupling constant of QCD decreases if the energy is increased – showed that quarks are forever confined. It was rewarded with the 2004 Nobel Prize in Physics, although a rigorous proof of quark confinement is still missing.

Gell-Mann did not just contribute to the physics of strong interactions. In 1979, along with Pierre Ramond and Richard Slansky, he wrote a paper discussing details of the “seesaw mechanism” – a theoretical proposal to account for the very small values of the neutrino masses introduced a couple of years earlier. After 1980 he also became interested in string theory. His wide-ranging interests in languages, and other areas beyond physics are also well documented.

I enjoyed working with Murray Gell-Mann. We had similar interests in physics, and we worked together until 1976 when I left Caltech and went to CERN. He visited often. In May 2019, during a trip to Los Alamos Laboratory, I was fortunate to have had the chance to visit Murray at his house in Santa Fe one last time. 

Further memories of Gell-Mann can be found at Gell-Mann’s multi-dimensional genius and Memories from Caltech.