Space goes quantum at Stony Brook

Like players on a stage, most forces act in a fixed, pre-existing space, but in Einstein’s classical theory of general relativity gravity is the dynamic shape of space. When classical forces enter the quantum arena the stage plays a new and more visible role: in its usual formulation quantum theory demands a ground state. The ground state is a fixed vacuum, above which excitations – which we can calculate and often measure to astonishing accuracy – propagate and interact. Because the vacuum is fixed it is not a surprise that for decades there was no convincing way to apply quantum principles to gravity.

Attempts were made to use the techniques of quantum field theory, which successfully quantizes light, to quantize Einstein’s theory. In this approach, just as light is described by a particle, the photon, gravity is described by a particle, the graviton. This is already a compromise as the graviton is a quantum ripple in a pre-assumed space that is not quantized. More drastically, the quantum field theory for the graviton fails because what should be small quantum corrections overwhelm the classical approximation, giving uncontrollable infinite modifications of the theory.

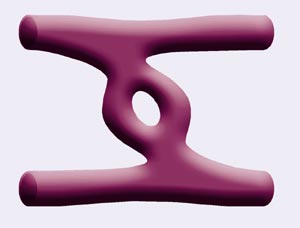
String theory solved part of this problem. In string theory the graviton is a string vibrating in one of its possible patterns, or “modes”. As the string moves through space it splits and rejoins itself (figure 1). These bifurcations and recombinations are the “stringy” quantum corrections, which are milder than those in quantum field theory and give rise to a quantum theory of gravity that is well defined and finite as a systematic expansion in the number of splittings. But the strings still move in space rather than being a part of it; they are quantized, while space itself remains stubbornly classical. Furthermore, it is not known if this expansion around Einstein’s classical theory can be summed to give a completely defined quantum theory.

Even before string theory John Wheeler suggested that at the Planck scale, the distance where the quantum corrections to gravity become large, the topology and geometry of space-time are unavoidably subject to quantum fluctuations (Wheeler 1964). This idea of a space-time quantum “foam” was explored by Stephen Hawking but has remained mysterious (Hawking 1978). New developments from an unexpected direction, however, have now given hints of an underlying, fundamentally quantum theory of strings that realizes these ideas: a mapping has been found to the theory of how crystals melt. In this picture classical geometry corresponds to a macroscopic crystal and quantum geometry to its underlying microscopic, atomic description.

These ideas grew out of discussions between a string theorist, Cumrun Vafa of Harvard, and two mathematicians, Nikolai Reshetikhin of UC Berkeley and Andrei Okounkov of the Institute for Advanced Study. They were brought together at the first of a series of interdisciplinary workshops held at the C N Yang Institute for Theoretical Physics and supported by the Simons Foundation at Stony Brook University in the summer of 2003.

The full theory of strings is still not understood well enough to formulate the problem of strings moving in a quantum space in complete generality. Instead, Okounkov, Reshetikhin and Vafa studied a part (or “sector”) of string theory called “topological string theory”. For concreteness, they focused on topological strings moving in a special class of spaces known as Calabi-Yau (CY) spaces.

Topological string theory is a simplification of the full theory of strings in which the motion of strings does not depend on the details of the space through which they move. As such it is mathematically more tractable than the full theory. At the same time, CY spaces are very interesting in string theory. In part this is because they are candidates for the as yet unobserved six dimensions that complement our familiar three dimensions of space and one of time. A widely discussed string-theory scenario is that the familiar four-dimensional space-time and a six-dimensional CY space combine to make up the 10-dimensional space-time that is required for the self-consistency of string theory.

Many interesting properties of topological string theory on CY spaces have already become known through the work of Vafa and collaborators over the past few years. In particular they have shown that quantum corrections can be computed. These corrections are given by the relative likelihoods that strings split and join as they move in the space. The potential for one string to split or join is measured by a number called the “string coupling”. This is actually a measure of the force between strings – in string theory forces are generated between two strings when one splits and one of its parts joins with the other. The larger the string coupling the more likely it is that this will happen and the stronger the force. These calculations are fine as long as the string coupling is small, but they become unmanageable when the coupling gets too large.

The crystal connection

At last summer’s Simons Workshop, Okounkov, Reshetikhin and Vafa realized that a formula describing the topological string splitting on a CY space also has a completely different interpretation involving a crystal composed of a regular array of idealized atoms (Okounkov et al. 2003). When they identified the temperature of the crystal with the inverse of the string coupling, the likelihood of an atom leaving the lattice became the same as that of a string splitting. Once this connection is made, the same formula that describes the splitting of the strings describes the melting of the crystal (figure 2).

At high temperatures the idealized crystal melts into a smooth surface with a well-defined shape. This surface is a two-dimensional portrait of a CY space, called a “projection” of the space (figure 3). At these temperatures the string coupling is small and topological strings can be described in terms of the calculable quantum corrections. However, as the string coupling and hence the force between strings increases, the strings split so often it is unclear how to compute their behaviour in string theory. But increasing string coupling means decreasing temperature, and at low temperatures the crystal theory comes to the rescue. The crystal becomes simple at low temperatures, with most atoms fixed in their positions in the lattice. This means that the smooth surface of the melted crystal is replaced by the discrete structure of the lattice. The CY space naturally becomes discrete.

This led Okounkov, Reshetikhin and Vafa to conclude that topological string theory and crystal theory are “dual” descriptions of a single underlying system valid for the whole range of weak and strong string coupling, or equivalently, high and low temperatures, respectively. In particular when the string coupling is small, quantum fluctuations appear only at scales much smaller than the natural size of the strings themselves, and the picture of smooth strings remains self-consistent.

The new picture that emerges from this duality is that of a “quantum” CY geometry. To understand what this means it is worth recalling that in a classical space of any kind each point is specified by a set of numbers, or co-ordinates. Examples of co-ordinates are the longitude and latitude of the Earth’s surface. In the quantum CY space the co-ordinates are no longer simple numbers to be specified at will. Rather they obey the Heisenberg uncertainty principle, which relates the position and momentum of a quantum particle. For the quantum CY spaces of Okounkov, Reshetikhin and Vafa’s dual description of topological string theory, the long-standing dream of replacing a smooth classical space with a discrete quantum substructure is thus realized. In this system the emergence of a classical geometry out of a quantum system can be clearly controlled and understood. As is shown in further work by Vafa et al., this gives an explicit and controllable picture of the Wheeler-Hawking notion of topological fluctuations – or “foam” – in space-time (Iqbal et al. 2003). The fluctuations of topology and geometry actually become the deep origin of strings. They extend rather than reduce the predictive power of the quantum theory of gravity.

Of course many challenges remain before a full theory of this kind can be realized. Chief among these is the extension of the picture from topological strings to full string theory. A possible path has been identified, however, suggesting that in string theory, as in Einstein’s gravity, the distinction between forces and the space in which they act melts away.