The promise and challenges of the Neutrino Factory.
The measurements of the electron- and muon-neutrino fluxes published by the Super-Kamiokande collaboration in 1998 marked a turning point in the history of particle physics. This team showed that fewer muon-neutrinos arrive at the surface of the Earth than are produced by cosmic-ray interactions in the upper atmosphere (atmospheric neutrinos). This in turn indicated evidence for neutrino oscillations, the phenomenon in which the flavour of the neutrino changes (oscillates) as the neutrino propagates through space and time. Since the publication of Super-Kamiokande’s seminal paper, the phenomenon of neutrino oscillations has been established through further measurements of atmospheric neutrinos, as well as of neutrinos and antineutrinos produced in the Sun, by nuclear reactors and by high-energy particle accelerators. It is arguably the most significant advance in particle physics of the past decade.
Extending the Standard Model
Neutrino oscillations imply that the Standard Model is incomplete and must be extended to include neutrino mass as well as mixing among the three neutrino flavours. The mechanism by which neutrino mass is generated is not known. An intriguing possibility is that the tiny neutrino mass is the result of physics at extremely high energy scales. Such a “see-saw” mechanism might also help to explain why neutrino mixing is so much stronger than the mixing among quarks. Mixing among three massive neutrinos admits the possibility that symmetry between matter and antimatter (CP-symmetry) is violated via the neutrino mixing matrix. Nonzero neutrino mass implies that lepton number must be used to distinguish a neutrino from an antineutrino. If lepton number is not conserved then a neutrino is indistinguishable from an antineutrino, i.e. the neutrino is a Majorana particle – a completely new state of matter. The determination of the properties of the neutrino, therefore, is fundamental to the development of particle physics.
These exciting new measurements imply that it may be possible to observe CP-violation in neutrino oscillations
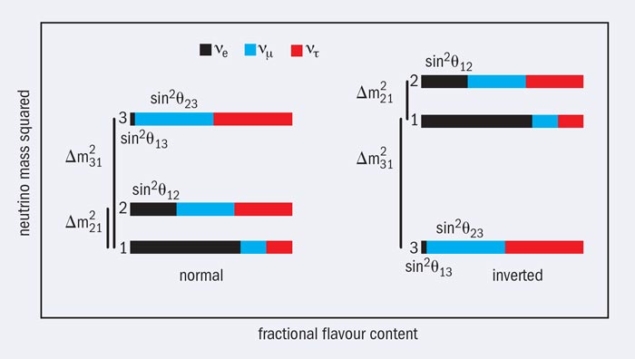
Neutrino oscillations are readily described by extending the Standard Model to include three neutrino-mass eigenstates, ν1, ν2 and ν3, such that the neutrino-flavour eigenstates, νe, νμ and ντ, are quantum-mechanical mixtures of the mass eigenstates (figure 1). Neutrino oscillations arise from the “beating” of the phase of the neutrino-mass eigenstates as a neutrino produced as an eigenstate of flavour propagates through space and time. The matrix by which the mass-basis is rotated into the flavour-basis is parameterized in terms of three mixing angles (θ12, θ23 and θ13) and one phase parameter (δ). If δ is nonzero (and not equal to π), then CP-violation in the neutrino sector will occur so long as θ13 > 0. Measurements of neutrino oscillations in vacuum can be used to determine the moduli of the mass-squared differences Δm231 = m23 – m21 and Δm221 = m22 – m21 and, with the aid of interactions with matter, also the sign.
The bulk of the measurements of neutrino oscillations to date have been collected using the dominant “disappearance” channels νe → νe and νμ → νμ. These data have yielded values for the three mixing angles, as well as for the magnitude of the mass-squared differences Δm231 and Δm221, and have shown that m2 > m1 (i.e. that Δm221 > 0). Last year, the T2K, MINOS and Double Chooz experiments presented evidence that θ13 may be greater than zero. Then, in March this year, the Daya Bay collaboration reported that sin22θ13 = 0.092 ± 0.016 (stat.) ± 0.005 (syst.), i.e. that sin22θ13 = 0 is excluded at 5.2 σ. The announcement was soon followed by the report of a similar result from the RENO experiment. These exciting new measurements imply that it may be possible to observe CP-violation in neutrino oscillations. The challenge for the neutrino community, therefore, is to refine the measurement of θ13 to determine the sign of Δm231 (the “mass hierarchy”), to discover CP-violation (if, indeed, it does occur) by measuring δ and to improve the accuracy with which θ23 is known.
Over the next few years, several experiments – MINOS, T2K, NOνA, Double Chooz, Daya Bay and RENO – will exploit the νμ→ νe and νe → νx channels to improve significantly the precision with which θ13 is known. The NOνA long-baseline experiment might also be able to determine the mass hierarchy. However, it is unlikely that either T2K or NOνA will be able to discover CP-violation, i.e. that δ ≠ 0 or π.
The Neutrino Factory
Neutrino oscillations also have implications well beyond the confines of particle physics. The possibility of CP-violation through the neutrino mixing matrix, combined with the possibility that the neutrino is a Majorana particle, makes it conceivable that the interactions of the neutrino led to the observed domination of matter over antimatter in the universe. The abundance of neutrinos in the universe is second only to that of photons. Even with a tiny mass, the neutrino may make a significant contribution to dark matter and thereby play an important role in determining the structure of the universe.
Such a breadth of impact justifies an ambitious, far-reaching experimental programme. Determining the nature of the neutrino – whether Majorana or Dirac – through the search for neutrinoless double-beta decay (2β0ν) is an important part of this programme. The absolute neutrino mass must also be determined either through observations of 2β0ν decay or from the measurement of the end-point of the electron spectrum in beta decay. Equally important is the accurate determination of the parameters that determine the properties of the neutrino. This requires intense, high-energy neutrino and antineutrino beams – precisely what the Neutrino Factory is designed to produce.
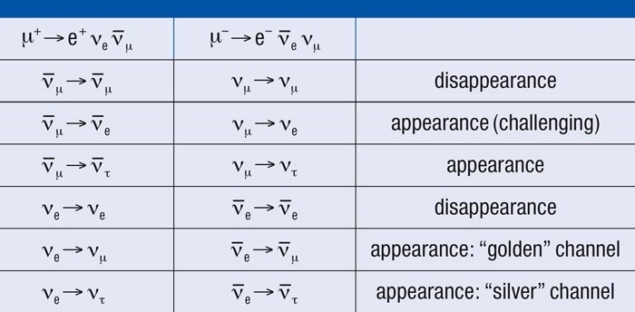
In the Neutrino Factory, beams of νe and νμ (νe,νμ) are produced from the decays of μ+ (μ–) circulating in a storage ring. High neutrino-energies can readily be achieved because the neutrinos carry away a substantial fraction of the energy of the muon. Time-dilation is beneficial, allowing sufficient time to produce a pure, collimated beam. The table above lists the oscillation channels that are available at the Neutrino Factory. Charged-current interactions induced by νe → νμ oscillations – the “gold channel” – produce muons that are opposite in charge to those produced by the νμ in the beam, so a magnetized detector is required. The additional capability to investigate the “silver” (νe → ντ) and “platinum” (νμ → νe) channels also makes the Neutrino Factory an excellent place to look for oscillation phenomena that are outside the standard three-neutrino mixing paradigm. It would be the ideal facility to serve the precision-era of neutrino-oscillation measurements.
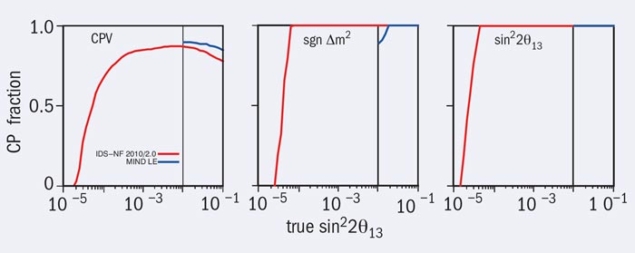
In 2011, the International Design Study for the Neutrino Factory (the IDS-NF) collaboration presented two options for the facility in its Interim Design Report (IDR) (Choubey et al. 2011). The first, optimized for discovery reach at small θ13 (sin22θ13 < 10–2), calls for two distant detectors, with baselines of 2500–5000 km and 7000–8000 km, and a stored-muon energy of 25 GeV. The second option, optimized for sensitivity at large θ13, requires a single detector at a distance of around 2000 km and a stored-muon beam with an energy of only 10 GeV. Figure 2 shows the discovery reach of the facility presented in terms of the fraction of all possible values of δ (the “CP fraction”) and plotted as a function of sin22θ13.
In the past few weeks, the Daya Bay and RENO collaborations have announced the first measurements of sin22θ13 with a value around 0.1. Figure 2 shows that at such a large value of θ13, excellent performance can be achieved using the “low-energy” option. At such a large value of θ13, the precision and discovery reach of a “low energy” Neutrino Factory is significantly better than the realistic alternatives (IDS-NF 2011).
Novel techniques
The IDS-NF baseline accelerator facility sketched in figure 3 provides a total of 1021 muon decays per year, split between the two distant neutrino detectors. The process of creating the muon beam begins with the bombardment of a pion-production target with a pulsed proton beam. The pions are captured in a solenoidal channel in which they decay to produce the muon beam. A sequence of accelerators is then used to manipulate and reduce (cool) the muon-beam phase space and to accelerate the muons to their final energy.
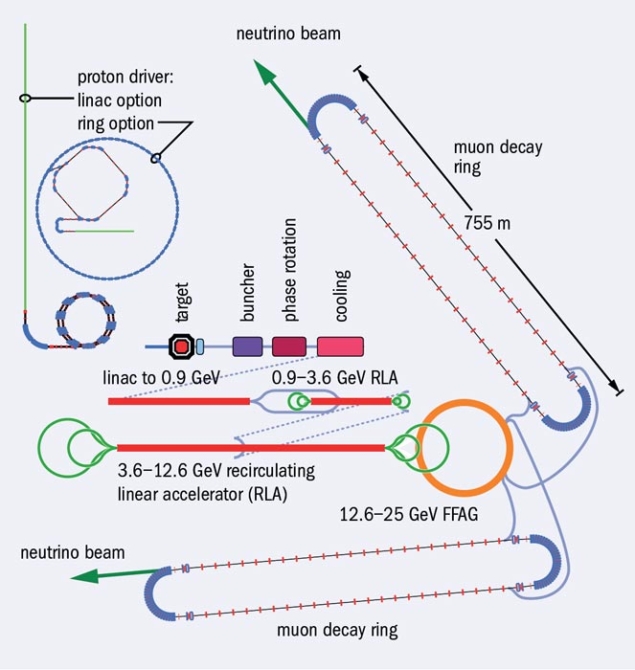
The muon’s short lifetime has required novel techniques to be developed to carry out these steps. Ionization cooling, the technique by which it is proposed to cool the muons, involves passing the beam through a material in which it loses energy through ionization and then re-accelerating it in the longitudinal direction to replace the lost energy. Muon acceleration will be carried out in a series of superconducting linear and recirculating linear accelerators. The final stage of acceleration, from 12.6 GeV to the stored-muon energy of 25 GeV, is provided by a fixed-field alternating-gradient (FFAG) accelerator. The baseline neutrino detector is a MINOS-like iron-scintillator sandwich calorimeter with a sampling fraction optimized for the Neutrino Factory beam. The baseline calls for a fiducial mass of 100 kilotonnes to be placed at the intermediate baseline and a detector of 50 kilotonnes at the magic baseline.
Image credit: S Kill/STFC.
Much of the Neutrino Factory facility, the accelerator complex and the neutrino detectors exploit state-of-the-art technologies. To achieve the ultimate performance (1021 muon decays per year) the IDS-NF baseline calls for: a proton-beam power of 4 MW, delivered at a repetition rate of 50 Hz in short (around 2 ns) bunches; a pion-production target capable of accepting the high proton-beam power; an ionization-cooling channel that increases the useful muon flux by a factor of around 2; and an FFAG to boost the beam energy rapidly to 25 GeV. R&D programmes that address each of these issues are underway. CERN, along with other proton-accelerator laboratories, is actively developing the technologies necessary to deliver multimega-watt, pulsed proton beams. The principle of a mercury-jet pion-production target was demonstrated by the MERIT experiment in 2008 that ran in the beamline of n_TOF, the neutron time-of-flight facility at CERN. The nonscaling FFAG accelerator EMMA (the Electron Model of Muon Acceleration, also known as the Electron Model of Many Applications) has been commissioned at the Daresbury Laboratory in the UK and used to demonstrate the “serpentine acceleration” characteristic of the nonscaling FFAG. The international Muon Ionization Cooling Experiment (MICE) at the Rutherford Appleton Laboratory will provide the engineering demonstration of the ionization-cooling technique (see box, previous page).
The Neutrino Factory is the facility of choice for the study of neutrino oscillations. It has excellent discovery reach and offers the best precision on the mixing parameters. The ability to vary the stored-muon energy and, perhaps the detector technology, gives the necessary flexibility to respond to developments in understanding neutrino physics and in the discovery of new phenomena. The R&D programme required to make the Neutrino Factory a reality will directly benefit the development of a muon collider and experiments that seek to discover charged lepton-flavour violation. The case for the Neutrino Factory as part of a comprehensive muon-physics programme is compelling indeed.
I gratefully acknowledge the help, advice, and support of my many colleagues within the IDS-NF, EUROnu and MICE collaborations and the Neutrino Factory community who have freely discussed their results with me and from whose work and results I have drawn freely.
BOX INSET
Cooling at MICE
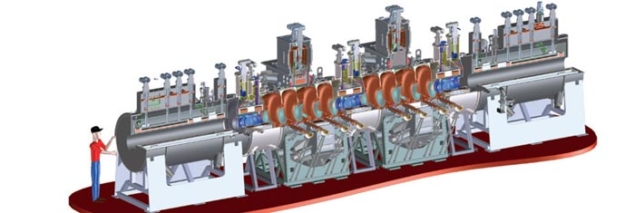
Image credit: A DeMello/LBNL.
MICE is a single-particle experiment in which the position and momentum of each muon is measured before it enters the MICE cooling channel and is measured again after it has left (Gregoire et al. 2003 and 2005). Muons with momenta between 140 MeV/c and 240 MeV/c, with normalized emittance between 2 πmm and 10 πmm, will be provided by a purpose-built beamline at the 800 MeV proton synchrotron, ISIS, at the Rutherford Appleton Laboratory.
The MICE cooling channel, a single lattice cell, comprises three 20-l volumes of liquid hydrogen and two short linac modules each consisting of four 201 MHz cavities. Beam transport is achieved by a series of superconducting solenoids: the “focus coils” focus the beam into the liquid-hydrogen absorbers, while a “coupling coil” surrounds each of the linac modules. A particle-identification system, with scintillator time-of-flight (TOF) hodoscopes and threshold Cherenkov counters, upstream of the cooling channel allows a pure muon beam to be selected. Downstream of the cooling channel, a final hodoscope and a calorimeter system allow muon decays to be identified. The calorimeter is composed of a lead-scintillator section, of a similar design to that of the KLOE detector at DAΦNE but with thinner lead foils, followed by a fully active scintillator detector (the electron-muon ranger) in which the muons are brought to rest.
Charged-particle tracking in MICE is provided by two solenoidal spectrometers that together determine the relative change in transverse emittance of the beam, which is expected to be approximately 10%, with a precision of ±1% (i.e. a 0.1% measurement of the change in absolute emittance). The trackers themselves are required to have high track-finding efficiency in the presence of background that is induced by X-rays produced in the RF cavities.
In the first “step” of the experiment, the muon beam for MICE has been characterized using the beamline instrumentation and the TOF, Cherenkov and lead-scintillator systems (figure 5). The results, which are being prepared for publication, show that the muon beam can provide the range of momentum and emittance required by MICE. The trackers and a prototype of the electron-muon ranger have been tested and shown to perform to specification. The cavities that make up the two short linac sections have been manufactured by Lawrence Berkeley National Laboratory (LBNL). The superconducting magnets required for the cooling channel are all under construction. By the end of 2012, the collaboration will commission the two spectrometer modules and the first liquid-hydrogen absorber and focus-coil module. This will allow preliminary studies of the ionization-cooling effect to be performed. The full MICE cooling cell will be constructed once the initial cooling studies are complete.





