ALICE investigates ‘snowballs in hell’

Résumé

L’omelette norvégienne d’ALICE

Il n’y a pas uniquement des hadrons ordinaires dans les débris des ” boules de feu ” produites par les collisions d’ions lourds effectuées à l’expérience ALICE auprès du LHC ; on y trouve aussi des objets composites, aux liaisons lâches, tels que deutérons et hypernoyaux légers et leurs antiparticules. Des études montrent que la production de ces particules, telle qu’elle peut être mesurée, concorde très bien avec les résultats calculés avec la même méthode que pour les hadrons, ce qui implique que les taux de production des objets à liaison lâche sont déterminés à la limite de phase entre le plasma quark-gluon de la boule de feu et un gaz hadronique. Comment est-ce possible ? La réponse est donnée par la thermodynamique.

The main goal of the ALICE experiment at the LHC is to produce and study the properties of matter as it existed in the first few microseconds after the Big Bang. Such matter consists of fermions and bosons, the fundamental entities of the Standard Model. Depending on the temperature, T, only particles with mass much less than T are copious. For T < 1 GeV, or about 10¹³ K, these are the u, d and s quarks and the gluons of the strong interactions. In addition, there are of course photons, leptons and neutrinos.

This “cosmic matter” can be produced in the laboratory by collisions at relativistic energies between very heavy atomic nuclei, such as lead at the LHC and gold at Brookhaven’s Relativistic Heavy Ion Collider (RHIC). In these collisions, a fireball is formed at (initial) temperatures up to 600 MeV, with a volume exceeding 1000 fm³ – about the volume of a lead nucleus – and with lifetimes exceeding 10 fm/c, about 3 × 10^–23 s. This space–time volume is macroscopic for strong interactions, but charged leptons, photons and neutrinos leave the fireball without interacting and play no part in the following discussion. (However, charged leptons and photons do have a role as penetrating probes of the produced matter.) Such deconfined cosmic matter is referred to as quark–gluon plasma (QGP) because its constituents carry colour and can roam freely within the volume of the fireball. At LHC energies, the QGP comprises, in addition to gluons, essentially equal numbers of quarks and antiquarks, i.e. it carries no net baryon number, as would also have been the case in the early universe.

The produced fireball expands and cools until it reaches the (pseudo-)critical temperature, T_c, of the deconfinement–confinement transition. Solving the strong-interaction equations on a discrete space–time lattice leads, in the most recent predictions, to T_c = 155±9 MeV. The yields of hadrons produced in central lead–lead (Pb–Pb) collisions at LHC energies and measured with the ALICE detector can indeed be quantitatively understood by assuming that they all originate from a thermalized state described with a grand-canonical thermal ensemble at T_chem = 156±2 MeV; the “chemical freeze-out” temperature T_chem is therefore very close to or coincides with T_c (see figure 1). While the overall agreement between data and model predictions is excellent, there is a 2.8σ discrepancy for (anti)protons, which is currently under scrutiny. Because the volume of the fireball is fixed by the number of particles produced, the temperature T_chem is the principal parameter determined in the grand-canonical analysis.

Such Pb–Pb collisions produce not only hadrons in the classical sense but also composite and even fragile objects such as light nuclei (d, t, ³He, ⁴He) and light Λ-hypernuclei, along with their antiparticles. Their measured yields decrease strongly with increasing (anti)baryon number – the penalty factor for each additional (anti)baryon is about 300 – hence (anti)⁴He production is a very rare process. Note that, because the fireball carries no net baryon number, the yields of the produced antiparticles closely coincide with the corresponding particle yields.

An interesting question is whether the yields of composite objects can be understood in the same grand-canonical scheme as discussed above, or whether such loosely bound objects follow a different systematics. The deuteron binding energy, for example, is only 2.23 MeV, and the energy needed to separate the Λ hyperon from a hypertriton nucleus – a bound state of a proton, neutron and Λ – is only about 130 keV, which is much smaller than the chemical freeze-out temperature, T_chem = 156 MeV.

Furthermore, the radii of such loosely bound objects are generally very large, even exceeding significantly the range of the nuclear force that binds them. The rms radius of the deuteron is 2.2 fm, for example. Even more dramatically, because of the molecular structure of the hypertriton ((p+n) + Λ), its rms radius, which in this case is the rms separation between the d nucleus and the Λ hyperon, is about 10 fm – that is, larger than the radius of the whole fireball.

Identification is the key

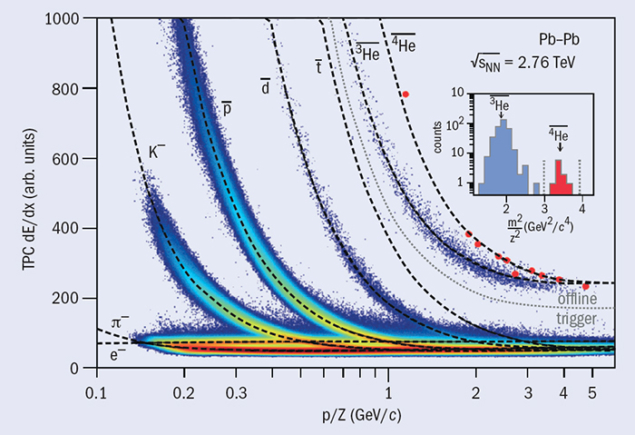
Before answering the question of how such exotic and fragile objects are produced, it is important to discuss how well such rare particles can be measured in the hostile environment of a Pb–Pb collision. In a central Pb–Pb collision at LHC energies, more than 15,000 charged particles are produced and up to 3000 go through the central barrel of the ALICE detector, making the task of tracking and identifying all of the different particle species quite a challenge. With ALICE, the identification of all of the produced particles and, in particular, the measurement of light nuclei and Λ-hypernuclei, is only possible because of the experiment’s excellent tracking and particle-identification capabilities via dE/dx and time-of-flight measurements. This is demonstrated in figure 2, which shows an event display from the ALICE time-projection chamber (TPC) for a central Pb–Pb collision. The highlighted black track corresponds to an identified anti⁴He track, implying that even such rare particles can be tracked with precision. Figure 3 shows the clean identification achieved for anti⁴He particles.

At first glance it is surprising that, as figure 1 shows, the measured yields of deuterons and hypertritons and their antiparticles agree very well with the yields calculated using the approach described above for hadrons at the same chemical freeze-out temperature, T_chem = 156 MeV. This implies that the yields of these loosely bound objects are determined at the phase boundary from the QGP to a hadron gas. How is this possible for such loosely bound objects whose sizes are much larger than the inter-particle separation at the time of chemical freeze-out?

To understand this, thermodynamics comes to the rescue. If there are no more inelastic collisions after chemical freeze-out, then the transition from the QGP to hadronic matter is followed by an isentropic expansion (i.e. with no change in entropy). Early studies of nucleus–nucleus collisions at the Berkeley Bevalac already showed that, for systems with isentropic expansion, the entropy/net-baryon is proportional to log(d/p), implying that the yield of deuterons and antideuterons is determined by the entropy in the hot phase of the fireball. The same mechanism is at play at LHC energies: in this way, the “snowballs” can survive “hell”, as the experimental data from the ALICE collaboration show.

These facts can be used to search for even more exotic states. ALICE has performed a search for two hypothetical strange dibaryon states. The first one is the H-dibaryon, which is a six-quark bound state of uuddss, first predicted by Robert Jaffe in a “bag-model” calculation in 1977. This early calculation led to a binding energy of 81 MeV. Recent non-perturbative QCD calculations (on the lattice) suggest either a loosely bound state or a resonant state above the ΛΛ threshold. The existence of double-Λ hypernuclei, such as the _ΛΛ⁴He, reduced the allowed binding energy to a maximum of 7.3 MeV, with the most preferred value around 1 MeV. The second hypothetical bound state investigated by ALICE is a possible Λn bound state.

The two searches are performed in central (0–10%) Pb–Pb collisions at √s_NN = 2.76 TeV in the decay modes H-dibaryon → Λpπ^– and Λn → dπ⁺. No signals are observed in either of the measured invariant-mass distributions, therefore setting upper limits for the production yields. These limits are well below the yields predicted using the grand-canonical scenario discussed above with T_chem = 156 MeV (see figure 1). In fact, the difference between the upper limit at 99% CL obtained for the Λn bound state is a factor of around 50 below the prediction, whereas the factor between the upper limit at 99% CL and the model prediction for the H-dibaryon is close to 25. Given the success of the model in predicting deuteron and hypertriton yields, it appears that the existence of such bound states is very unlikely.

With the LHC’s Run 2, which has just started, and much more so with the upgraded ALICE apparatus in LHC Run 3, it is expected that ALICE can measure hypernuclei with still higher masses, such as _Λ⁴H and _ΛΛ⁴He and the corresponding antiparticles. These would be the highest-mass antihypernuclei ever observed. In addition, the hypertriton measurement will be extended in the three-body decay _Λ³H → d + p + π^–. The much higher statistics expected will also allow more detailed measurements, such as determination of the ⁴He transverse-momentum spectrum. In addition, searches are underway for other hypothetical bound states such as Λnn or other exotic di-baryons.

In summary, the success in describing the production of different hadrons and the yields of loosely bound objects with the same temperature, T, provides strong evidence for isentropic expansion after the transition from the QGP to a hadron gas. This scenario naturally explains the observed yields for loosely bound objects. On the other hand, the upper limits obtained for the H-dibaryon and the Λn bound state are well below the model prediction using the same temperature, T = 156 MeV, casting serious doubts on their existence.

The ALICE data on light (anti)nucleus production in pp, p–Pb and Pb–Pb collisions shows that very loosely bound objects are produced with significant yields for all systems, with the thermodynamic limit reached for the Pb–Pb system. The measured yields are expected to increase with beam energy similar to the way that the overall multiplicity density does. This implies significant production of antideuterons from high-energy cosmic rays, with potential consequences for searches for dark matter. Their yields can be well predicted within the scenario described here.