After 30 years, a theory is within reach for high-T superconductors.

Image credit: H Barnard/CERN.
Superconductivity is perhaps the most remarkable manifestation of quantum physics on the macroscopic scale. Discovered in 1911 by Kamerlingh Onnes, it preoccupied the most prominent physicists of the 20th century and remains at the forefront of condensed-matter physics today. The interest is partly driven by potential applications – superconductivity at room temperature would surely revolutionise technology – but to a large extent it reflects an intellectual fascination. Many ideas that emerged from the study of superconductivity, such as the generation of a photon mass in a superconductor, were later extended to other fields of physics, famously serving as paradigms to explain the generation of a Higgs mass of the electroweak W and Z gauge bosons in particle physics.
Put simply, superconductivity is the ability of a system of fermions to carry electric current without dissipation. Normally, fermions such as electrons scatter off any obstacle, including each other. But if they find a way to form bound pairs, these pairs may condense into a macroscopic state with a non-dissipative current. Quantum mechanics is the only way to explain this phenomenon, but it took 46 years after the discovery of superconductivity for Bardeen, Cooper and Schrieffer (BCS) to develop a verifiable theory. Winning the 1972 Nobel Prize in Physics for their efforts, they figured out that the exchange of phonons leads to an effective attraction between pairs of electrons of opposite momentum if the electron energy is less than the characteristic phonon energy (figure 1). Although electrons still repel each other, the effective Coulomb interaction becomes smaller at such frequencies (in a manner opposite to asymptotic freedom in high-energy physics). If the reduction is strong enough, the phonon-induced electron–electron attraction wins over Coulomb repulsion and the total interaction becomes attractive. There is no threshold for the magnitude of the attraction because low-energy fermions live at the boundary of the Fermi sea, in which case an arbitrary weak attraction is enough to create bound states of fermions at some critical temperature, Tc.
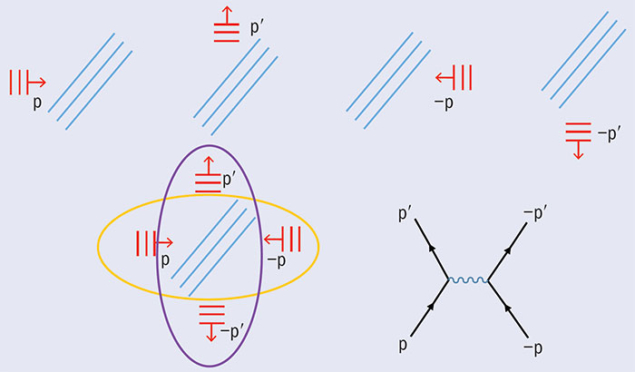
The formation of bound states, called Cooper pairs, is one necessary ingredient for superconductivity. The other is for the pairs to condense, or more specifically to acquire a common phase corresponding to a single macroscopic wave function. Within BCS theory, pair formation and locking of the phases of the pairs occur simultaneously at the same Tc, while in more recent strong-coupling theories bound pairs exist above this temperature. The common phase of the pairs can have an arbitrary value, and the fact that the system chooses a particular one below Tc is a manifestation of spontaneous symmetry breaking. The phase coherence throughout the sample is the most important physical aspect of the superconducting state below Tc, as it can give rise to a “supercurrent” that flows without resistance. Superconductivity can also be viewed as an emergent phenomenon.
The BCS electron–phonon mechanism of superconductivity has since been successfully applied to explain pairing in a large variety of materials
While BCS theory was a big success, it is a mean-field theory, which neglects fluctuations. To really trust that the electron–phonon mechanism was correct, it was necessary to develop theoretical tools based on Green functions and field-theory methods, and to move beyond weak coupling. The BCS electron–phonon mechanism of superconductivity has since been successfully applied to explain pairing in a large variety of materials (figure 2), from simple mercury and aluminium to the niobium-titanium and niobium-tin alloys used in the magnets for the Large Hadron Collider (LHC), in addition to the recently discovered sulphur hydrides, which become superconductors at a temperature of around 200 K under high pressure. But the discovery of high-temperature superconductors drove condensed-matter theorists to explore new explanations for the superconducting state.
Unconventional superconductors
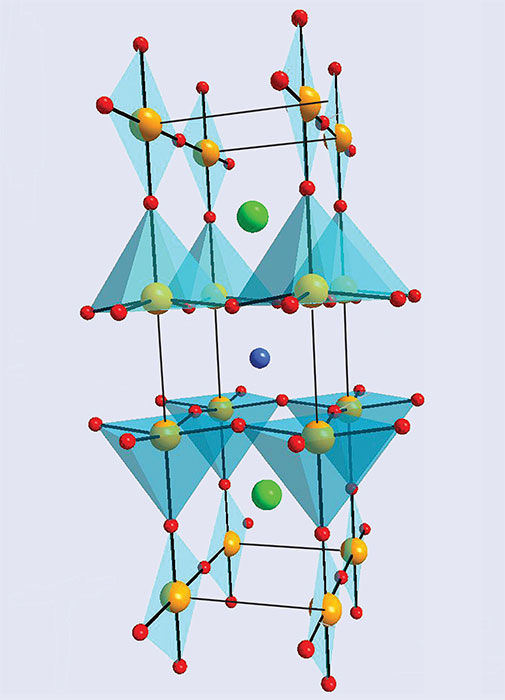
In the early 1980s, when the record critical temperature for superconductors was of the order 20 K, the dream of a superconductor that works at liquid-nitrogen temperatures (77 K) seemed far off. In 1986, however, Bednorz and Müller made the breakthrough discovery of superconductivity in La1−xBaxCuO4 with Tc of around 40 K. Shortly after, a material with a similar copper-oxide-based structure with Tc of 92 K was discovered. These copper-based superconductors, known as cuprates, have a distinctive structure comprising weakly coupled layers made of copper and oxygen. In all the cuprates, the building blocks for superconductivity are the CuO2 planes, with the other atoms providing a charge reservoir that either supplies additional electrons to the layers or takes electrons out to leave additional hole states (figure 3).
From a theoretical perspective, the high Tc of the cuprates is only one important aspect of their behaviour. More intriguing is what mechanism binds the fermions into pairs. The vast majority of researchers working in this area think that, unlike low-temperature superconductors, phonons are not responsible. The most compelling reason is that the cuprates possess “unconventional” symmetry of the pair wave function. Namely, in all known phonon-mediated superconductors, the pair wave function has s-wave symmetry, or in other words, its angular dependence is isotropic. For the cuprates, it was proven in the early 1990s that the pair wave function changes sign under rotation by 90°, leading to an excitation spectrum that has zeros at particular points on the Fermi surface. Such symmetry is often called “d-wave”. This is the first symmetry beyond s-wave that is allowed by the antisymmetric nature of the electron wave functions when the total spin of the pair is zero. The observation of a d-wave symmetry in the cuprates was extremely surprising because, unlike s-wave pairs, d-wave Cooper pairs can potentially be broken by impurities.
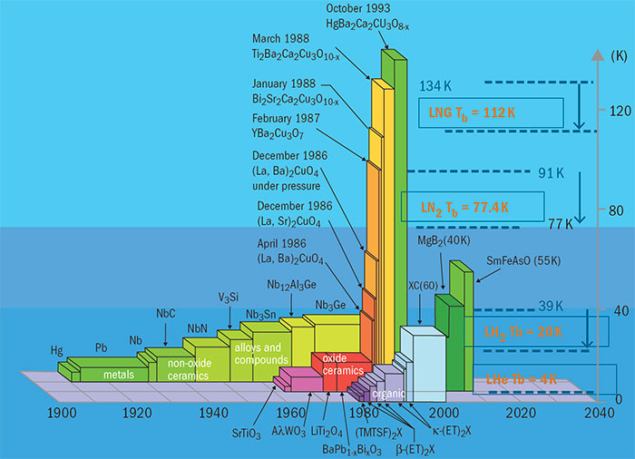
Image credit: B Glowacki.
The cuprates hold the record for the highest Tc for materials with an unconventional pair wave-function symmetry: 133 K in mercury-based HgBa2Ca2Cu3O8 at ambient pressure. They were not, however, the first materials of this kind: a “heavy fermion” superconductor CeCu2Si2 discovered in 1979 by Steglich, and an organic superconductor discovered by Jerome the following year, also had an unconventional pair symmetry. After the discovery of cuprates, a set of unconventional iron-based superconductors was discovered with Tc up to 60 K in bulk systems, followed by the discovery of superconductivity with an even higher Tc in a monolayer of FeSe. But even low-Tc, unconventional materials can be interesting. For example, some experiments suggest that Cooper pairs in Sr2RuO4 have total spin-one and p-wave symmetry, leading to the intriguing possibility that they can support edge modes that are Majorana particles, which have potential applications in quantum computing.
If phonon-mediated electron–electron interactions are ineffective for the pairing in unconventional superconductors, then what binds fermions together? The only other possibility is a nominally repulsive electron–electron interaction, but for this to allow pairing, the electrons must screen their own Coulomb repulsion to make it effectively attractive in at least one pairing channel (e.g. d-wave). Interestingly, quantum mechanics actually allows such schizophrenic behaviour of electrons: a d-wave component of a screened Coulomb interaction becomes attractive in certain cases.
Cuprate conundrums
There are several families of high-temperature cuprate superconductors. Some, like LaSrCuO, YBaCuO and BSCCO, show superconductivity upon hole doping; others, like NdCeCuO, show superconductivity upon electron doping. The phase diagram of a representative cuprate contains regions of superconductivity, regions of magnetic order, and a region (called the pseudogap) where Tc decreases but the system’s behaviour above Tc is qualitatively different from that in an ordinary metal (figure 4). At zero doping, standard solid-state physics says that the system should be a metal, but experiments show that it is an insulator. This is taken as an indication that the effective interaction between electrons is large, and such an interaction-driven insulator is called a Mott insulator. Upon doping, some states become empty and the system eventually recovers metallic behaviour. A Mott insulator at zero doping has another interesting property: spins of localised electrons order antiferromagnetically. Upon doping, the long-range antiferromagnetic order quickly disappears, while short-range magnetic correlations survive.
Since the superconducting region of the phase diagram is sandwiched between the Mott and metallic regimes, there are two ways to think about HTS: either it emerges upon doping of a Mott insulator (if one departs from zero doping), or it emerges from a metal with increased antiferromagnetic correlations if one departs from larger dopings. Even though it was known before the discovery of high-temperature superconductors that antiferromagnetically mediated interaction is attractive in the d-wave channel, it took time to develop various computational approaches, and today the computed value of Tc is in the range consistent with experiments. At smaller dopings, a more reliable approach is to start from a Mott insulator. This approach also gives d-wave superconductivity, with the value of Tc most likely determined by phase fluctuations and decreasing as a function of decreased doping. Because both approaches give d-wave superconductivity with comparable values of Tc, the majority of researchers believe that the mechanism of superconductivity in the cuprates is understood, at least qualitatively.
A more subtle issue is how to explain the so-called pseudogap phase in hole-doped cuprates (figure 4). Here, the system is neither magnetic nor superconducting, yet it displays properties that clearly distinguish it from a normal, even strongly correlated metal. One natural idea, pioneered by Philip Anderson, is that the pseudogap phase is a precursor to a Mott insulator that contains a soup of local singlet pairs of fermions: superconductivity arises if the phases of all singlet pairs are ordered, whereas antiferromagnetism arises if the system develops a mixture of spin singlets and spin triplets. Several theoretical approaches, most notably dynamical mean-field theory, have been developed to quantitatively describe the precursors to a Mott insulator.
The understanding of the pseudogap as the phase where electron states progressively get localised, leading to a reduction of Tc, is accepted by many in the HTS community. Yet, new experimental results show that the pseudogap phase in hole-doped cuprates may actually be a state with a broken symmetry, or at least becomes unstable to such a state at a lower temperature. Evidence has been reported for the breaking of time-reversal, inversion and lattice rotational symmetry. Improved instrumentation in recent years also led to the discovery of a charge-density wave and pair-density wave order in the phase diagram and perhaps even loop-current order. Many of us believe that the additional orders observed in the pseudogap phase are relevant to the understanding of the full phase diagram, but that these do not change the two key pillars of our understanding: superconductivity is mediated by short-range magnetic excitations, and the reduction of Tc at smaller dopings is due the existence of a Mott insulator near zero doping.
Woodstock physics

Participants at a special session of the 1987 March meeting of the American Physical Society in New York devoted to the newly discovered high-temperature superconductors. The hastily organised session, which later became known as the “Woodstock of Physics” lasted from the early evening to 3.30 a.m. the following morning, with 51 presenters and more than 1800 physicists in attendance. Bednorz and Müller received the Nobel prize in December 1987, one year after the discovery, which was the fastest award in the Nobel’s history.
Why cuprates still matter
The cuprates have motivated incredible advances in instrumentation and experimental techniques, with 1000-fold increases in accuracy in many cases. On the theoretical side, they have also led to the development of new methods to deal with strong interactions – dynamical mean-field theory and various metallic quantum-critical theories are examples. These experimental and theoretical methods have found their way into the study of other materials and are adding new chapters to standard solid-state physics books. Some of them may even one day find their way into other fields, such as strongly interacting quark–gluon matter. We can now theoretically understand a host of the phenomena in high-temperature superconductors, but there are still some important points to clarify, such as the mysterious linear temperature dependence of the resistivity.
The community is coming together to solve these remaining issues. Yet, the cynical view of the cuprate problem is that it lacks an obvious small parameter, and hence a universally accepted theory – the analogue of BCS – will never be developed. While it is true that serendipity will always have its place in science, we believe that the key criterion for “the theory” of the cuprates should not be a perfect quantitative agreement with experiments (even though this is still a desirable objective). Rather, a theory of cuprates should be judged by its ability to explain both superconductivity and a host of concomitant phenomena, such as the pseudogap, and its ability to provide design principles for new superconductors. Indeed, this is precisely the approach that allowed the recent discovery of the highest-Tc superconductor to date: hydrogen sulphide. At present, powerful algorithms and supercomputers allow us to predict quite accurately the properties of materials before they are synthesised. For strongly correlated materials such as the cuprates, these calculations profit from physical insight and vice versa.
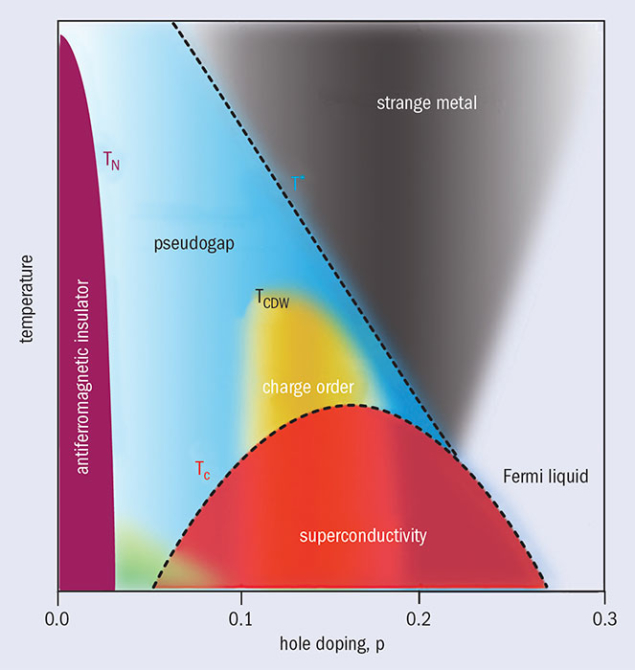
Image credit: Inna Vishik, UCDavis.
From a broader perspective, studies of HTS have led to renewed thinking about perturbative and non-perturbative approaches to physics. Physicists like to understand particles or waves and how they interact with each other, like we do in classical mechanics, and perturbation theory is the tool that takes us there – QED is a great example that works because the fine-structure constant is small. In a single-band solid where interactions are not too strong, it is natural to think of superconductivity as being mediated by, for example, the exchange of antiferromagnetic spin fluctuations. When interactions are so strong that the wave functions become extremely entangled, it still makes sense to look at the internal dynamics of a Cooper pair to check whether one can detect traces of spin, charge or even orbital fluctuations. At the same time, perturbation theory in the usual sense does not work. Instead, we have to rely more heavily on large-scale computer calculations, variational approaches and effective theories. The question of what “binds” fermions into a Cooper pair still makes sense in this new paradigm, but the answer is often more nuanced than in a weak coupling limit.
Many challenges are left in the HTS field, but progress is rapid and there is much more consensus now than there was even a few years ago. Finally, after 30 years, it seems we are closing in on a theoretical understanding of this both useful and fascinating macroscopic quantum state.
CERN puts high-temperature superconductors to use
A few years ago, triggered by conceptual studies for a post-LHC collider, CERN launched a collaboration to explore the use of high-temperature superconductors (HTS) for accelerator magnets. In 2013 CERN partnered with a European particle accelerator R&D project called EuCARD-2 to develop a HTS insert for a 20 T magnet. The project came to an end in April this year, with CERN having built an HTS demonstration magnet based on an “aligned-block” concept for which coil-winding and quench-detection technology had to be developed. Called Feather2, the magnet has a field of 3 T based on low-performance REBCO (rare-earth barium-copper-oxide) tape. The next magnet, based on high-performance REBCO tape, will approach a stand-alone field of 8 T. Then, once it is placed inside the aperture of the 13 T “Fresca2” magnet, the field should go beyond 20 T.
Now the collaborative European spirit of EuCARD-2 lives on in the ARIES project (Accelerator Research and Innovation for European Science and Society), which kicked off at CERN in May. ARIES brings together 41 participants from 18 European countries, including seven industrial partners, to help bring down the cost of the conductor, and is co-funded via a contribution of €10 million from the European Commission.
In addition, CERN is developing HTS-based transfer lines to feed the new superconducting magnets of the High Luminosity LHC based on magnesium diboride (MgB2), which can be operated in helium gas at temperatures of up to around 30 K and must be flexible enough to allow the power converters to be installed hundreds of metres away from the accelerator. The relatively low cost of MgB2 led CERN’s Amalia Ballarino to enter a collaboration with industry, which resulted in a method to produce MgB2 in wire form for the first time. The team has since achieved record currents that reached 20 kA at a temperature above 20 K, thereby proving that MgB2 technology is a viable solution for long-distance power transmission. The new superconducting lines could also find applications in the Future Circular Collider initiative.
• Matthew Chalmers, CERN