From BCS to the LHC

It was a little odd for me, a physicist whose work has been mainly on the theory of elementary particles, to be invited to speak at a meeting of condensed-matter physicists celebrating a great achievement in their field. It is not only that there is a difference in the subjects that we explore. There are deep differences in our aims, in the kinds of satisfaction that we hope to get from our work.

Condensed-matter physicists are often motivated to deal with phenomena because the phenomena themselves are intrinsically so interesting. Who would not be fascinated by weird things, such as superconductivity, superfluidity, or the quantum Hall effect? On the other hand, I don’t think that elementary-particle physicists are generally very excited by the phenomena they study. The particles themselves are practically featureless, every electron looking tediously just like every other electron.

Another aim of condensed-matter physics is to make discoveries that are useful. In contrast, although elementary-particle physicists like to point to the technological spin-offs from elementary-particle experimentation, and these are real, this is not the reason that we want these experiments to be done, and the knowledge gained by these experiments has no foreseeable practical applications.

Most of us do elementary-particle physics neither because of the intrinsic interestingness of the phenomena that we study, nor because of the practical importance of what we learn, but because we are pursuing a reductionist vision. All of the properties of ordinary matter are what they are because of the principles of atomic and nuclear physics, which are what they are because of the rules of the Standard Model of elementary particles, which are what they are because…well, we don’t know, this is the reductionist frontier, which we are currently exploring.

I think that the single most important thing accomplished by the theory of John Bardeen, Leon Cooper, and Robert Schrieffer (BCS) was to show that superconductivity is not part of the reductionist frontier (Bardeen et al. 1957). Before BCS this was not so clear. For instance, in 1933 Walter Meissner raised the question of whether electric currents in superconductors are carried by the known charged particles, electrons and ions. The great thing that Bardeen, Cooper, and Schrieffer showed was that no new particles or forces had to be introduced to understand superconductivity. According to a book on superconductivity that Cooper showed me, many physicists were even disappointed that “superconductivity should, on the atomistic scale, be revealed as nothing more than a footling small interaction between electrons and lattice vibrations”. (Mendelssohn 1966).

His testimony was so scrupulously honest that I think it helped the SSC more than it hurt it.

The claim of elementary-particle physicists to be leading the exploration of the reductionist frontier has at times produced resentment among condensed-matter physicists. (This was not helped by a distinguished particle theorist, who was fond of referring to condensed-matter physics as “squalid state physics”.) This resentment surfaced during the debate over the funding of the Superconducting Super Collider (SSC). I remember that Phil Anderson and I testified in the same Senate committee hearing on the issue, he against the SSC and I for it. His testimony was so scrupulously honest that I think it helped the SSC more than it hurt it. What really did hurt was a statement opposing the SSC by a condensed-matter physicist who happened at the time to be the president of the American Physical Society. As everyone knows, the SSC project was cancelled, and now we are waiting for the LHC at CERN to get us moving ahead again in elementary-particle physics.

During the SSC debate, Anderson and other condensed-matter physicists repeatedly made the point that the knowledge gained in elementary-particle physics would be unlikely to help them to understand emergent phenomena like superconductivity. This is certainly true, but I think beside the point, because that is not why we are studying elementary particles; our aim is to push back the reductive frontier, to get closer to whatever simple and general theory accounts for everything in nature. It could be said equally that the knowledge gained by condensed-matter physics is unlikely to give us any direct help in constructing more fundamental theories of nature.

So what business does a particle physicist like me have at a celebration of the BCS theory? (I have written just one paper about superconductivity, a paper of monumental unimportance, which was treated by the condensed-matter community with the indifference it deserved.) Condensed-matter physics and particle physics are relevant to each other, despite everything I have said. This is because, although the knowledge gained in elementary-particle physics is not likely to be useful to condensed-matter physicists, or vice versa, experience shows that the ideas developed in one field can prove very useful in the other. Sometimes these ideas become transformed in translation, so that they even pick up a renewed value to the field in which they were first conceived.

The example that concerns me is an idea that elementary-particle physicists learnt from condensed-matter theory – specifically from the BCS theory. It is the idea of spontaneous symmetry breaking.

Spontaneous symmetry breaking

In particle physics we are particularly interested in the symmetries of the laws of nature. One of these symmetries is invariance of the laws of nature under the symmetry group of three-dimensional rotations, or in other words, invariance of the laws that we discover under changes in the orientation of our measuring apparatus.

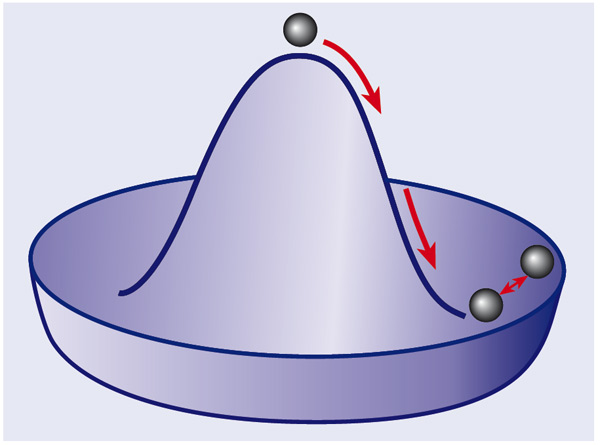
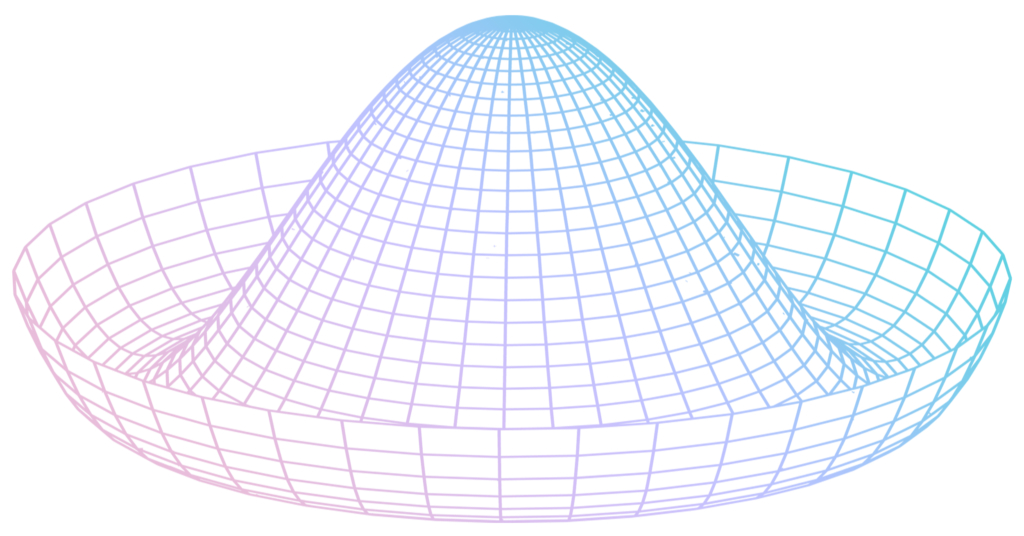
When a physical system does not exhibit all the symmetries of the laws by which it is governed, we say that these symmetries are spontaneously broken. A very familiar example is spontaneous magnetization. The laws governing the atoms in a magnet are perfectly invariant under three-dimensional rotations, but at temperatures below a critical value, the spins of these atoms spontaneously line up in some direction, producing a magnetic field. In this case, and as often happens, a subgroup is left invariant: the two-dimensional group of rotations around the direction of magnetization.

Now to the point. A superconductor of any kind is nothing more or less than a material in which a particular symmetry of the laws of nature, electromagnetic gauge invariance, is spontaneously broken. This is true of high-temperature superconductors, as well as the more familiar superconductors studied by BCS. The symmetry group here is the group of two-dimensional rotations. These rotations act on a two-dimensional vector, whose two components are the real and imaginary parts of the electron field, the quantum mechanical operator that in quantum field theories of matter destroys electrons. The rotation angle of the broken symmetry group can vary with location in the superconductor, and then the symmetry transformations also affect the electromagnetic potentials, a point to which I will return.

The symmetry breaking in a superconductor leaves unbroken a rotation by 180°

The symmetry breaking in a superconductor leaves unbroken a rotation by 180°, which simply changes the sign of the electron field. In consequence of this spontaneous symmetry breaking, products of any even number of electron fields have non-vanishing expectation values in a superconductor, though a single electron field does not. All of the dramatic exact properties of superconductors – zero electrical resistance, the expelling of magnetic fields from superconductors known as the Meissner effect, the quantization of magnetic flux through a thick superconducting ring, and the Josephson formula for the frequency of the AC current at a junction between two superconductors with different voltages – follow from the assumption that electromagnetic gauge invariance is broken in this way, with no need to inquire into the mechanism by which the symmetry is broken.

Condensed-matter physicists often trace these phenomena to the appearance of an “order parameter”, the non-vanishing mean value of the product of two electron fields, but I think this is misleading. There is nothing special about two electron fields; one might just as well take the order parameter as the product of three electron fields and the complex conjugate of another electron field. The important thing is the broken symmetry, and the unbroken subgroup.

It may then come as a surprise that spontaneous symmetry breaking is mentioned nowhere in the seminal paper of Bardeen, Cooper and Schrieffer. Their paper describes a mechanism by which electromagnetic gauge invariance is in fact broken, but they derived the properties of superconductors from their dynamical model, not from the mere fact of broken symmetry. I am not saying that Bardeen, Cooper, and Schrieffer did not know of this spontaneous symmetry breaking. Indeed, there was already a large literature on the apparent violation of gauge invariance in phenomenological theories of superconductivity, the fact that the electric current produced by an electromagnetic field in a superconductor depends on a quantity known as the vector potential, which is not gauge invariant. But their attention was focused on the details of the dynamics rather than the symmetry breaking.

This is not just a matter of style. As BCS themselves made clear, their dynamical model was based on an approximation, that a pair of electrons interact only when the magnitude of their momenta is very close to a certain value, known as the Fermi surface. This leaves a question: How can you understand the exact properties of superconductors, like exactly zero resistance and exact flux quantization, on the basis of an approximate dynamical theory? It is only the argument from exact symmetry principles that can fully explain the remarkable exact properties of superconductors.

Though spontaneous symmetry breaking was not emphasized in the BCS paper, the recognition of this phenomenon produced a revolution in elementary-particle physics. The reason is that (with certain qualification, to which I will return), whenever a symmetry is spontaneously broken, there must exist excitations of the system with a frequency that vanishes in the limit of large wavelength. In elementary-particle physics, this means a particle of zero mass.

The first clue to this general result was a remark in a 1960 paper by Yoichiro Nambu, that just such collective excitations in superconductors play a crucial role in reconciling the apparent failure of gauge invariance in a superconductor with the exact gauge invariance of the underlying theory governing matter and electromagnetism. Nambu speculated that these collective excitations are a necessary consequence of this exact gauge invariance.

Nambu put this idea to good use in particle physics

A little later, Nambu put this idea to good use in particle physics. In nuclear beta decay an electron and neutrino (or their antiparticles) are created by currents of two different kinds flowing in the nucleus, known as vector and axial vector currents. It was known that the vector current was conserved, in the same sense as the ordinary electric current. Could the axial current also be conserved?

The conservation of a current is usually a symptom of some symmetry of the underlying theory, and holds whether or not the symmetry is spontaneously broken. For the ordinary electric current, this symmetry is electromagnetic gauge invariance. Likewise, the vector current in beta decay is conserved because of the isotopic spin symmetry of nuclear physics. One could easily imagine several different symmetries, of a sort known as chiral symmetries, that would entail a conserved axial vector current. However, it seemed that any such chiral symmetries would imply either that the nucleon mass is zero, which is certainly not true, or that there must exist a triplet of massless strongly interacting particles of zero spin and negative parity, which isn’t true either. These two possibilities simply correspond to the two possibilities that the symmetry, whatever it is, either is not, or is, spontaneously broken, not just in some material like a superconductor, but even in empty space.

Nambu proposed that there is indeed such a symmetry, and it is spontaneously broken in empty space, but the symmetry in addition to being spontaneously broken is not exact to begin with, so the particle of zero spin and negative parity required by the symmetry breaking is not massless, only much lighter than other strongly interacting particles. This light particle, he recognized, is nothing but the pion, the lightest and first discovered of all the mesons. In a subsequent paper with Giovanni Jona-Lasinio, Nambu presented an illustrative theory in which, with some drastic approximations, a suitable chiral symmetry was found to be spontaneously broken, and in consequence the light pion appeared as a bound state of a nucleon and an antinucleon.

So far, there was no proof that broken exact symmetries always entail exactly massless particles, just a number of examples of approximate calculations in specific theories. In 1961 Jeffrey Goldstone gave some more examples of this sort, and a hand-waving proof that this was a general result. Such massless particles are today known as Goldstone bosons, or Nambu–Goldstone bosons. Soon after, Goldstone, Abdus Salam and I made this into a rigorous and apparently quite general theorem.

Cosmological fluctuations

This theorem has applications in many branches of physics. One is cosmology. You may know that today the observation of fluctuations in the cosmic microwave background are being used to set constraints on the nature of the exponential expansion, known as inflation, that is widely believed to have preceded the radiation-dominated Big Bang. But there is a problem here. In between the end of inflation and the time that the microwave background that we observe was emitted, there intervened a number of events that are not at all understood: the heating of the universe after inflation, the production of baryons, the decoupling of cold dark matter, and so on. So how is it possible to learn anything about inflation by studying radiation that was emitted long after inflation, when we don’t understand what happened in between? The reason that we can get away with this is that the cosmological fluctuations now being studied are of a type, known as adiabatic, that can be regarded as the Goldstone excitations required by a symmetry, related to general co-ordinate invariance, that is spontaneously broken by the space–time geometry. The physical wavelengths of these cosmological fluctuations were stretched out by inflation so much that they were very large during the epochs when things were happening that we don’t understand, so they then had zero frequency, which means that the amplitude of these fluctuations was not changing, so that the value of the amplitude relatively close to the present tells us what it was during inflation.

Werner Heisenberg continued to believe this into the 1970s

But in particle physics, this theorem was at first seen as a disappointing result. There was a crazy idea going around, which I have to admit that at first I shared, that somehow the phenomenon of spontaneous symmetry breaking would explain why the symmetries being discovered in strong-interaction physics were not exact. Werner Heisenberg continued to believe this into the 1970s, when everyone else had learned better.

The prediction of new massless particles, which were ruled out experimentally, seemed in the early 1960s to close off this hope. But it was a false hope anyway. Except under special circumstances, a spontaneously broken symmetry does not look at all like an approximate unbroken symmetry; it manifests itself in the masslessness of spin-zero bosons, and in details of their interactions. Today we understand approximate symmetries such as isospin and chiral invariance as consequences of the fact that some quark masses, for some unknown reason, happen to be relatively small.

Though based on a false hope, this disappointment had an important consequence. Peter Higgs, Robert Brout and François Englert, and Gerald Guralnik, Dick Hagen and Tom Kibble were all led to look for, and then found, an exception to the theorem of Goldstone, Salam and me. The exception applies to theories in which the underlying physics is invariant under local symmetries, symmetries whose transformations, like electromagnetic gauge transformations, can vary from place to place in space and time. (This is in contrast with the chiral symmetry associated with the axial vector current of beta decay, which applies only when the symmetry transformations are the same throughout space–time.) For each local symmetry there must exist a vector field, like the electromagnetic field, whose quanta would be massless if the symmetry was not spontaneously broken. The quanta of each such field are particles with helicity (the component of angular momentum in the direction of motion) equal in natural units to +1 or –1. But if the symmetry is spontaneously broken, these two helicity states join up with the helicity-zero state of the Goldstone boson to form the three helicity states of a massive particle of spin one. Thus, as shown by Higgs, Brout and Englert, and Guralnik, Hagen and Kibble, when a local symmetry is spontaneously broken, neither the vector particles with which the symmetry is associated nor the Nambu–Goldstone particles produced by the symmetry breaking have zero mass.

This was actually argued earlier by Anderson, on the basis of the example provided by the BCS theory. But the BCS theory is non-relativistic, and the Lorentz invariance that is characteristic of special relativity had played a crucial role in the theorem of Goldstone, Salam and me, so Anderson’s argument was generally ignored by particle theorists. In fact, Anderson was right: the reason for the exception noted by Higgs et al. is that it is not possible to quantize a theory with a local symmetry in a way that preserves both manifest Lorentz invariance and the usual rules of quantum mechanics, including the requirement that probabilities be positive. In fact, there are two ways to quantize theories with local symmetries: one way that preserves positive probabilities but loses manifest Lorentz invariance, and another that preserves manifest Lorentz invariance but seems to lose positive probabilities, so in fact these theories actually do respect both Lorentz invariance and positive probabilities; they just don’t respect our theorem.

Effective field theories

The appearance of mass for the quanta of the vector bosons in a theory with local symmetry re-opened an old proposal of Chen Ning Yang and Robert Mills, that the strong interactions might be produced by the vector bosons associated with some sort of local symmetry, more complicated than the familiar electromagnetic gauge invariance. This possibility was specially emphasized by Brout and Englert. It took a few years for this idea to mature into a specific theory, which then turned out not to be a theory of strong interactions.

Perhaps the delay was because the earlier idea of Nambu, that the pion was the nearly massless boson associated with an approximate chiral symmetry that is not a local symmetry, was looking better and better. I was very much involved in this work, and would love to go into the details, but that would take me too far from BCS. I’ll just say that, from the effort to understand processes involving any number of low-energy pions beyond the lowest order of perturbation theory, we became comfortable with the use of effective field theories in particle physics. The mathematical techniques developed in this work in particle physics were then used by Joseph Polchinski and others to justify the approximations made by BCS in their work on superconductivity.

The story of the physical application of spontaneously broken local symmetries has often been told, by me and others, and I don’t want to take much time on it here, but I can’t leave it out altogether because I want to make a point about it that will take me back to the BCS theory. Briefly, in 1967 I went back to the idea of a theory of strong interactions based on a spontaneously broken local symmetry group, and right away, I ran into a problem: the subgroup consisting of ordinary isospin transformations is not spontaneously broken, so there would be a massless vector particle associated with these transformations with the spin and charges of the ρ meson. This, of course, was in gross disagreement with observation; the ρ meson is neither massless nor particularly light.

The theory requires a massless vector particle, but it is not the ρ meson, it is the photon

Then it occurred to me that I was working on the wrong problem. What I should have been working on were the weak nuclear interactions, like beta decay. There was just one natural choice for an appropriate local symmetry, and when I looked back at the literature I found that the symmetry group I had decided on was one that had already been proposed in 1961 by Sheldon Glashow, though not in the context of an exact spontaneously broken local symmetry. (I found later that the same group had also been considered by Salam and John Ward.) Even though it was now exact, the symmetry when spontaneously broken would yield massive vector particles, the charged W particles that had been the subject of theoretical speculation for decades, and a neutral particle, which I called the Z particle, to mediate a “neutral current” weak interaction, which had not yet been observed. The same symmetry breaking also gives mass to the electron and other leptons, and in a simple extension of the theory, to the quarks. This symmetry group contained electromagnetic gauge invariance, and since this subgroup is clearly not spontaneously broken (except in superconductors), the theory requires a massless vector particle, but it is not the ρ meson, it is the photon, the quantum of light. This theory, which became known as the electroweak theory, was also proposed independently in 1968 by Salam.

The mathematical consistency of the theory, which Salam and I had suggested but not proved, was shown in 1971 by Gerard ‘t Hooft; neutral current weak interactions were found in 1973; and the W and Z particles were discovered at CERN a decade later. Their detailed properties are just those expected according to the electroweak theory.

There was (and still is) one outstanding issue: just how is the local electroweak symmetry broken? In the BCS theory, the spontaneous breakdown of electromagnetic gauge invariance arises because of attractive forces between electrons near the Fermi surface. These forces don’t have to be strong; the symmetry is broken however weak these forces may be. But this feature occurs only because of the existence of a Fermi surface, so in this respect the BCS theory is a misleading guide for particle physics. In the absence of a Fermi surface, dynamical spontaneous symmetry breakdown requires the action of strong forces. There are no forces acting on the known quarks and leptons that are anywhere strong enough to produce the observed breakdown of the local electroweak symmetry dynamically, so Salam and I did not assume a dynamical symmetry breakdown; instead we introduced elementary scalar fields into the theory, whose vacuum expectation values in the classical approximation would break the symmetry.

This has an important consequence. The only elementary scalar quanta in the theory that are eliminated by spontaneous symmetry breaking are those that become the helicity-zero states of the W and Z vector particles. The other elementary scalars appear as physical particles, now generically known as Higgs bosons. It is the Higgs boson predicted by the electroweak theory of Salam and me that will be the primary target of the new LHC accelerator, to be completed at CERN sometime in 2008.

But there is another possibility, suggested independently in the late 1970s by Leonard Susskind and me. The electroweak symmetry might be broken dynamically after all, as in the BCS theory. For this to be possible, it is necessary to introduce new extra-strong forces, known as technicolour forces, that act on new particles, other than the known quarks and leptons. With these assumptions, it is easy to get the right masses for the W and Z particles and large masses for all the new particles, but there are serious difficulties in giving masses to the ordinary quarks and leptons. Still, it is possible that experiments at the LHC will not find Higgs bosons, but instead will find a great variety of heavy new particles associated with technicolour. Either way, the LHC is likely to settle the question of how the electroweak symmetry is broken.

It would have been nice if we could have settled this question by calculation alone, without the need for the LHC, in the way that Bardeen, Cooper and Schrieffer were able to find how electromagnetic gauge invariance is broken in a superconductor by applying the known principles of electromagnetism. But that is just the price we in particle physics have to pay for working in a field whose underlying principles are not yet known.

• This article is based on the talk given by Steven Weinberg at BCS@50, held on 10–13 October 2007 at the University of Illinois at Urbana–Champaign to celebrate the 50th anniversary of the BCS paper. For more about the conference see www.conferences.uiuc.edu/bcs50/.