A look at some of the work behind two well known equations.

Image credit: D Diakonov.
Most particle physicists will be familiar with two famous abbreviations, DGLAP and BFKL, which are synonymous with calculations of high-energy, strong-interaction scattering processes, in particular nowadays at HERA, the Tevatron and most recently, the LHC. The Dokshitzer-Gribov-Lipatov-Alterelli-Parisi (DGLAP) equation and the Balitsky-Fadin-Kuraev-Lipatov (BFKL) equation together form the basis of current understanding of high-energy scattering in quantum chromodynamics (QCD), the theory of strong interactions. The celebration this year of the 70th birthday of Lev Lipatov, whose name appears as the common factor, provides a good occasion to look back at some of the work that led to the two equations and its roots in the theoretical particle physics of the 1960s.
Quantum field theory (QFT) lies at the heart of QCD. Fifty years ago, however, theoreticians were generally disappointed in their attempts to apply QFT to strong interactions. They began to develop methods to circumvent traditional QFT by studying the unitarity and analyticity constraints on scattering amplitudes, and extending Tullio Regge’s ideas on complex angular momenta to relativistic theory. It was around this time that the group in Leningrad led by Vladimir Gribov, which included Lipatov, began to take a lead in these studies.
Quantum electrodynamics (QED) provided the theoretical laboratory to check the new ideas of particle “reggeization”. In several pioneering papers Gribov, Lipatov and co-authors developed the leading-logarithm approximation to processes at high-energies; this later played a key role in perturbative QCD for strong interactions (Gorshkov et al. 1966). Using QED as an example, they demonstrated that QFT leads to a total cross-section that does not decrease with energy – the first example of what is known as Pomeron exchange. Moreover, they checked and confirmed the main features of Reggeon field theory in the particular case of QED.

By the end of the 1960s, experiments at SLAC had revealed Bjorken scaling in deep inelastic lepton-hadron scattering. This led Richard Feynman and James Bjorken to introduce nucleon constituents – partons – that later turned out to be nothing other than quarks, antiquarks and gluons. Gribov became interested in finding out if Bjorken scaling could be reproduced in QFT. As examples he studied both a fermion theory with a pseudoscalar coupling and QED, in the kinematic conditions where there is a large momentum-transfer, Q2, to the fermion. The task was to select and sum all leading Feynman diagrams that give rise to the logarithmically enhanced (α log Q2)n contributions to the cross section, at fixed values of the Bjorken variable x=Q2/(s+Q2) between zero and unity, where s is the invariant energy of the reaction.
At some point Lipatov joined Gribov in the project and together they studied not only deep inelastic scattering but also the inclusive annihilation of e+e– to a particle, h, in two field-theoretical models, one of which was QED. They showed that in a renormalizable QFT, the structure functions must violate Bjorken scaling (Gribov and Lipatov 1971). They obtained relations between structure functions that describe deep inelastic scattering and those that describe jet fragmentation in e+e– annihilation – the Gribov-Lipatov reciprocity relations. It is interesting to note that this work appeared at a time before experiments had either detected any violation in Bjorken scaling or observed any rise with momentum transfer of the transverse momenta in “hard” hadronic reactions, as would follow from a renormalizable field theory. This paradox led to continuous and sometimes heated discussions in the new Theory Division of the Leningrad (now Petersburg) Nuclear Physics Institute (PNPI) in Gatchina.
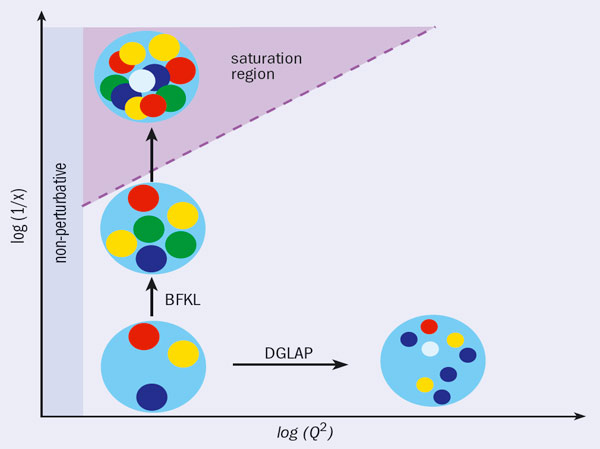
Somewhat later, Lipatov reformulated the Gribov-Lipatov results for QED in the form of the evolution equations for parton densities (Lipatov 1974). This differed from the real thing, QCD, only by colour factors and by the absence of the gluon-to-gluon-splitting kernel, which was later provided independently by Yuri Dokshitzer at PNPI, and by Guido Altarelli and Giorgio Parisi, then at Ecole Normale Superieure and IHES, Bures-sur-Yvette, respectively (Dokshitzer 1977, Altarelli and Parisi 1977). Today the Gribov-Lipatov-Dokshitzer-Altarelli-Parisi (DGLAP) evolution equations are the basis for all of the phenomenological approaches that are used to describe hadron interactions at short distances.
The more general evolution equation for quasi-partonic operators that Lipatov and his co-authors obtained allowed them to consider more complicated reactions, including high-twist operators and polarization phenomena in hard hadronic processes.
Lipatov went on to show that the gauge vector boson in Yang-Mills theory is “reggeized”: with radiative corrections included, the vector boson becomes a moving pole in the complex angular momentum plane near j=1. In QCD, however, this pole is not directly observable by itself because it corresponds to colour exchange. More meaningful is an exchange of two or more reggeized gluons, which leads to “colourless” exchange in the t-channel, either with vacuum quantum numbers (when it is called a Pomeron) or non-vacuum ones (when it is called an “odderon”). Lipatov and his collaborators showed that the Pomeron corresponds not to a pole, but to a cut in the plane of complex angular momentum.
A different approach
The case of high-energy scattering required a different approach. In this case, in contrast to the DGLAP approach – which sums up higher-order αs contributions enhanced by the logarithm of virtuality, ln Q2 – contributions enhanced by the logarithm of energy, ln s, or by the logarithm of a small momentum fraction, x, carried by gluons, become important. The leading-log contributions of the type (αsln(1/x))n are summed up by the famous Balitsky-Fadin-Kuraev-Lipatov (BFKL) equation (Kuraev et al. 1977, Balitsky and Lipatov 1978). Compared with DGLAP, this is a more complicated problem because the BFKL equation actually includes contributions from operators of higher twists.
In its general form the BFKL equation describes not only the high-energy behaviour of cross-sections but also the amplitudes at non-zero momentum transfer. Lipatov discovered beautiful symmetries in this equation, which enabled him to find solutions in terms of the conformal-symmetric eigenfunctions. This completed the construction of the “bare Pomeron in QCD”, a fundamental entity of high-energy physics (Lipatov 1986). An interesting new property of this bare Pomeron (which was not known in the old reggeon field theory) is the diffusion of the emitted particles in ln kt space.
Later, in the 1990s, Lipatov together with Victor Fadin calculated the next-to-leading-order corrections to the BFKL equation, obtaining the “BFKL Pomeron in the next-to-leading approximation” (Fadin and Lipatov 1998). Independently, this was also done by Marcello Ciafaloni and Gianni Camici in Florence (Ciafaloni and Camici 1998). Lipatov also studied higher-order amplitudes with an arbitrary number of gluons exchanged in the t-channel and, in particular, described odderon exchange in perturbative QCD. The significance of this work was, however, much greater. It led to the discovery of the connection between high-energy scattering and the exactly solvable two-dimensional field-theoretical models (Lipatov 1994).
More recently Lipatov has taken these ideas into the hot, new field in theoretical physics: the anti-de Sitter/conformal-field theory correspondence (ADS/CFT) – a hypothesis put forward by Juan Maldacena in 1997. This states that there is a correspondence – a duality – in the description of the maximally supersymmetric N=4 modification of QCD from the standard field-theory side and, from the “gravity” side, in the spectrum of a string moving in a peculiar curved anti-de Sitter background – a seemingly unrelated problem. However, Lipatov’s experience and deep understanding of re-summed perturbation theory has enabled him to move quickly into this new territory where he has developed and tested new ideas, considering first the BFKL and DGLAP equations in the N=4 theory and computing the anomalous dimensions of various operators. The high symmetry of this theory, in contrast to standard QCD, allows calculations to be made at unprecedented high orders and the results then compared with the “dual” predictions of string theory. It also facilitates finding the integrable structures in the theory (Lipatov 2009).
In this work, Lipatov has collaborated with many people, including Vitaly Velizhanin, Alexander Kotikov, Jochen Bartels, Matthias Staudacher and others. Their work is establishing the duality hypothesis almost beyond doubt. This opens a new horizon in studying QFT at strong couplings – something that no one would have dreamt of 50 years ago.
• The author thanks Victor Fadin and Mikhail Ryskin for helpful comments.